ISSN 0253-2778
CN 34-1054/N
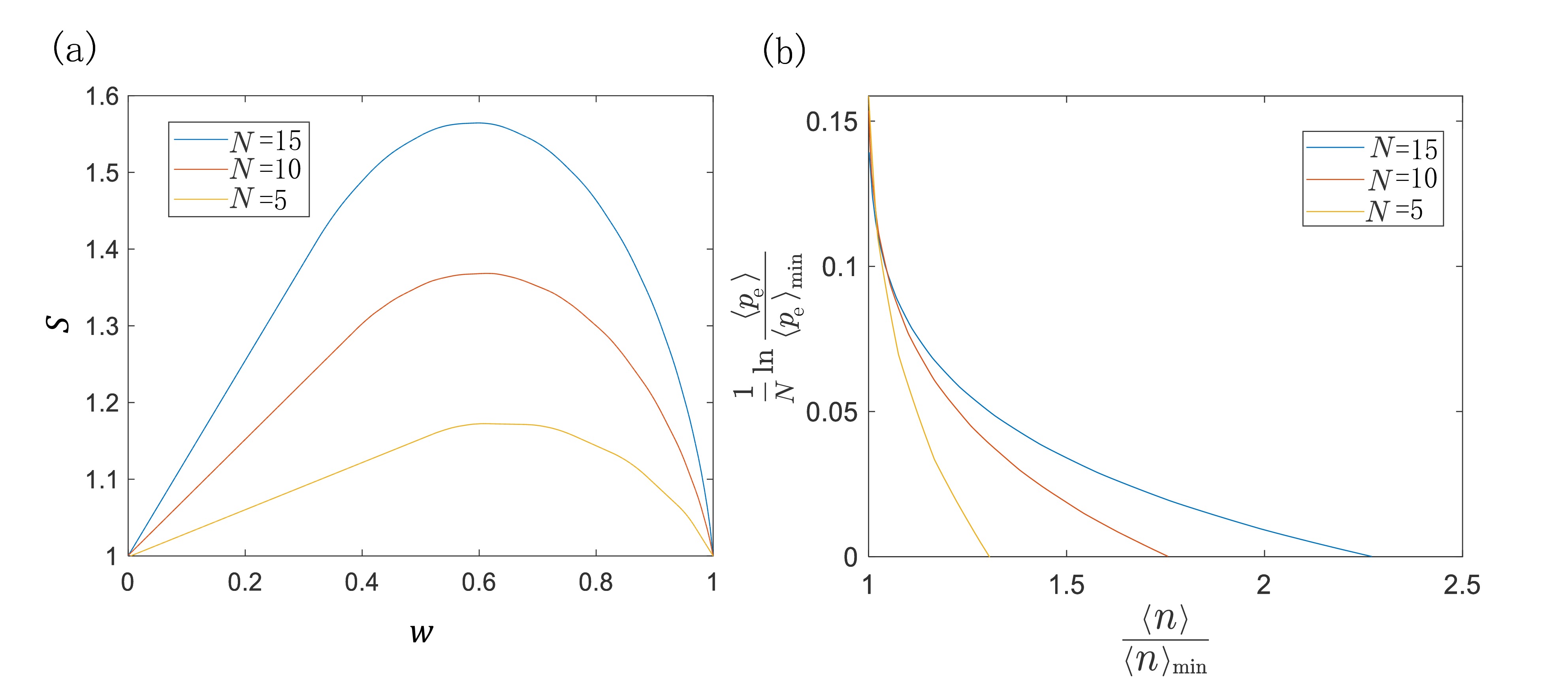
Extracting more information and saving quantum resources are two main aims for quantum measurements. However, the optimization of strategies for these two objectives varies when discriminating between quantum states
Balancing the minimum error rate and copy consumption in optimal quantum state discrimination.
| [1] |
Bennett C H. Quantum cryptography using any two nonorthogonal states. Physical Review Letters, 1992, 68: 3121–3124. DOI: 10.1103/PhysRevLett.68.3121
|
| [2] |
Gisin N, Ribordy G, Tittel W, et al. Quantum cryptography. Reviews of Modern Physics, 2002, 74: 145. DOI: 10.1103/RevModPhys.74.145
|
| [3] |
van Enk S J. Unambiguous state discrimination of coherent states with linear optics: Application to quantum cryptography. Physical Review A, 2002, 66: 042313. DOI: 10.1103/PhysRevA.66.042313
|
| [4] |
Knill E, Laflamme R, Zurek W H. Resilient quantum computation: Error models and thresholds. Proceedings of the Royal Society of London Series A: Mathematical, Physical and Engineering Sciences, 1998, 454: 365–384. DOI: 10.1098/rspa.1998.0166
|
| [5] |
Aharonov D, Ben-Or M. Fault tolerant quantum computation with constant error. In: Proceedings of the Twenty-ninth Annual ACM Symposium on Theory of Computing. New York: ACM, 1997 : 176–188.
|
| [6] |
Bennett C H, DiVincenzo D P. Quantum information and computation. Nature, 2000, 404: 247–255. DOI: 10.1038/35005001
|
| [7] |
Helstrom C W. Quantum detection and estimation theory. Journal of Statistical Physics, 1969, 1: 231–252. DOI: 10.1007/BF01007479
|
| [8] |
Higgins B L, Booth B M, Doherty A C, et al. Mixed state discrimination using optimal control. Physical Review Letters, 2009, 103: 220503. DOI: 10.1103/PhysRevLett.103.220503
|
| [9] |
Calsamiglia J, de Vicente J I, Muñoz-Tapia R, et al. Local discrimination of mixed states. Physical Review Letters, 2010, 105: 080504. DOI: 10.1103/PhysRevLett.105.080504
|
| [10] |
Higgins B L, Doherty A C, Bartlett S D, et al. Multiple-copy state discrimination: Thinking globally, acting locally. Physical Review A, 2011, 83: 052314. DOI: 10.1103/PhysRevA.83.052314
|
| [11] |
Wiseman H M, Milburn G J. Quantum Measurement and Control. Cambridge, UK: Cambridge University Press, 2009 .
|
| [12] |
Acín A, Bagan E, Baig M, et al. Multiple-copy two-state discrimination with individual measurements. Physical Review A, 2005, 71: 032338. DOI: 10.1103/PhysRevA.71.032338
|
| [13] |
Brody D, Meister B. Minimum decision cost for quantum ensembles. Physical Review Letters, 1996, 76: 1–5. DOI: 10.1103/PhysRevLett.76.1
|
| [14] |
Slussarenko S, Weston M M, Li J G, et al. Quantum state discrimination using the minimum average number of copies. Physical Review Letters, 2017, 118: 030502. DOI: 10.1103/PhysRevLett.118.030502
|
| [15] |
Martínez Vargas E, Hirche C, Sentís G, et al. Quantum sequential hypothesis testing. Physical Review Letters, 2021, 126: 180502. DOI: 10.1103/PhysRevLett.126.180502
|
| [16] |
Li Y, Tan V Y F, Tomamichel M. Optimal adaptive strategies for sequential quantum hypothesis testing. Communications in Mathematical Physics, 2022, 392: 993–1027. DOI: 10.1007/s00220-022-04362-5
|
| [17] |
Renes J M, Blume-Kohout R, Scott A J, et al. Symmetric informationally complete quantum measurements. Journal of Mathematical Physics, 2004, 45: 2171–2180. DOI: 10.1063/1.1737053
|
| [18] |
Conlon L O, Eilenberger F, Lam P K, et al. Discriminating mixed qubit states with collective measurements. Communication Physics, 2023, 6: 337. DOI: 10.1038/s42005-023-01454-z
|
| [19] |
Peres A, Wootters W K. Optimal detection of quantum information. Physical Review Letters, 1991, 66: 1119–1122. DOI: 10.1103/PhysRevLett.66.1119
|
| [20] |
Xu F, Zhang X M, Xu L, et al. Experimental quantum target detection approaching the fundamental Helstrom limit. Physical Review Letters, 2021, 127: 040504. DOI: 10.1103/PhysRevLett.127.040504
|
| [21] |
Cook R L, Martin P J, Geremia J M. Optical coherent state discrimination using a closed-loop quantum measurement. Nature, 2007, 446: 774–777. DOI: 10.1038/nature05655
|
| [22] |
Tian B, Yan W, Hou Z, et al. Minimum-consumption discrimination of quantum states via globally optimal adaptive measurements. Physical Review Letters, 2024, 132: 110801. DOI: 10.1103/PhysRevLett.132.110801
|