ISSN 0253-2778
CN 34-1054/N
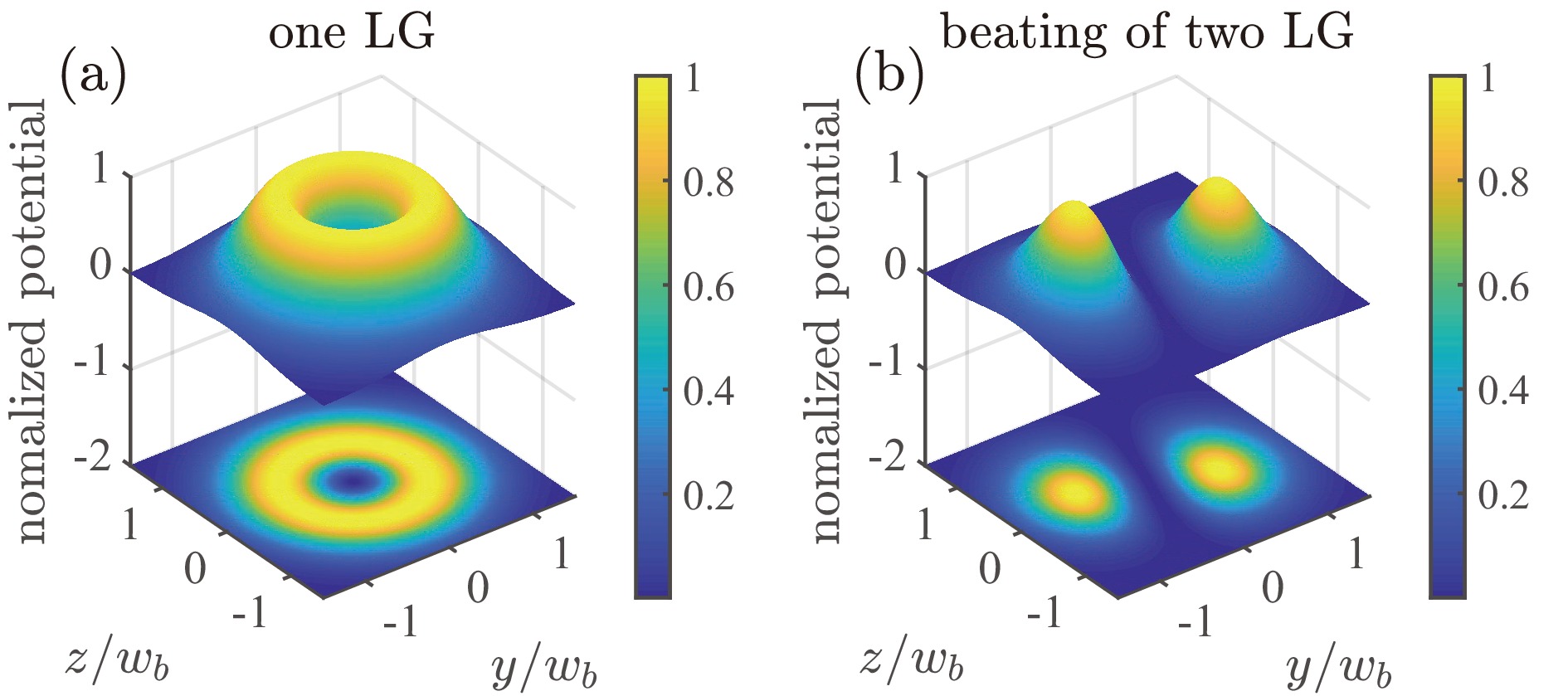
We present the results of twisted plasma waves driven by twisted ponderomotive force. With the beating of two, co-propagating, Laguerre-Gaussian (LG) orbital angular momentum (OAM) laser pulses with different frequencies and also different twist indices, we can obtain the twisted ponderomotive force. Three-dimensional particle-in-cell simulations are used to demonstrate the twisted plasma waves driven by lasers. The twisted plasma waves have an electron density perturbation with a helical rotating structure. Different from the predictions of the linear fluid theory, the simulation results show a nonlinear rotating current and a static axial magnetic field. Along with the rotating current is the axial OAM carried by particles in the twisted plasma waves. A detailed theoretical analysis of twisted plasma waves is also given.
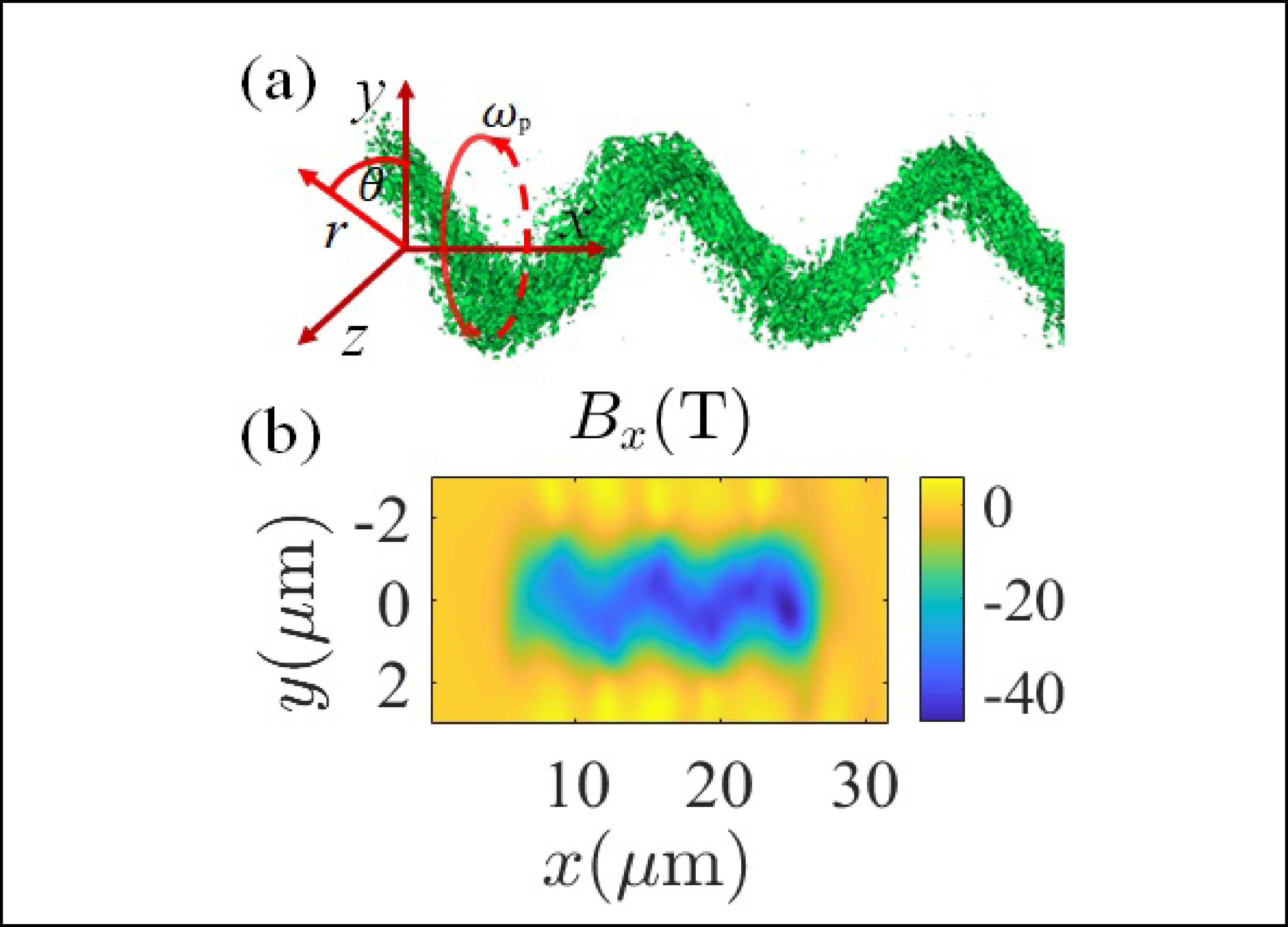
Twisted plasma waves driven by twisted ponderomotive force. (a) A 3D view of the electron density deviation in the plasma wave driven by twisted ponderomotive force, simulated using the EPOCH PIC code. (b) The distribution of longitudinal magnetic field at z = 0.
Figure
1.
Structure of the ponderomotive potential
Figure
3.
PIC results of transverse profile of (a) electron density perturbation
| [1] |
Esarey E, Schroeder C B, Leemans W P. Physics of laser-driven plasma-based electron accelerators. Reviews of Modern Physics, 2009, 81: 1229–1285. DOI: 10.1103/revmodphys.81.1229
|
| [2] |
Ali S, Davies J R, Mendonca J T. Inverse Faraday effect with linearly polarized laser pulses. Physical Review Letters, 2010, 105: 035001. DOI: 10.1103/physrevlett.105.035001
|
| [3] |
Haines M G. Generation of an axial magnetic field from photon spin. Physical Review Letters, 2001, 87: 135005. DOI: 10.1103/physrevlett.87.135005
|
| [4] |
Najmudin Z, Tatarakis M, Pukhov A, et al. Measurements of the inverse Faraday effect from relativistic laser interactions with an underdense plasma. Physical Review Letters, 2001, 87: 215004. DOI: 10.1103/physrevlett.87.215004
|
| [5] |
Sheng Z M, Meyer-ter-Vehn J. Inverse Faraday effect and propagation of circularly polarized intense laser beams in plasmas. Physical Review E, Statistical Physics, Plasmas, Fluids, and Related Interdisciplinary Topics, 1996, 54: 1833–1842. DOI: 10.1103/physreve.54.1833
|
| [6] |
Allen L, Beijersbergen M W, Spreeuw R J, et al. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Physical Review A, Atomic, Molecular, and Optical Physics, 1992, 45: 8185–8189. DOI: 10.1103/physreva.45.8185
|
| [7] |
Yao A M, Padgett M J. Orbital angular momentum: Origins, behavior and applications. Advances in Optics and Photonics, 2011, 3: 161. DOI: 10.1364/aop.3.000161
|
| [8] |
Shi Y, Shen B, Zhang L, et al. Light fan driven by a relativistic laser pulse. Physical Review Letters, 2014, 112: 235001. DOI: 10.1103/PhysRevLett.112.235001
|
| [9] |
Vieira J, Trines R M, Alves E P, et al. High orbital angular momentum harmonic generation. Physical Review Letters, 2016, 117: 265001. DOI: 10.1103/PhysRevLett.117.265001
|
| [10] |
Zhang L, Shen B, Zhang X, et al. Deflection of a reflected intense vortex laser beam. Physical Review Letters, 2016, 117: 113904. DOI: 10.1103/PhysRevLett.117.113904
|
| [11] |
Zhang X, Shen B, Shi Y, et al. Generation of intense high-order vortex harmonics. Physical Review Letters, 2015, 114: 173901. DOI: 10.1103/PhysRevLett.114.173901
|
| [12] |
Vieira J, Mendonça J T. Nonlinear laser driven donut wakefields for positron and electron acceleration. Physical Review Letters, 2014, 112: 215001. DOI: 10.1103/PhysRevLett.112.215001
|
| [13] |
Wang W, Shen B, Zhang X, et al. Hollow screw-like drill in plasma using an intense Laguerre–Gaussian laser. Scientific Reports, 2015, 5: 8274. DOI: 10.1038/srep08274
|
| [14] |
Zhang X, Shen B, Zhang L, et al. Proton acceleration in underdense plasma by ultraintense Laguerre-Gaussian laser pulse. New Journal of Physics, 2014, 16: 123051. DOI: 10.1088/1367-2630/16/12/123051
|
| [15] |
Vieira J, Mendonça J T, Quéré F. Optical control of the topology of laser-plasma accelerators. Physical Review Letters, 2018, 121: 054801. DOI: 10.1103/PhysRevLett.121.054801
|
| [16] |
Longman A, Fedosejevs R. Mode conversion efficiency to Laguerre-Gaussian OAM modes using spiral phase optics. Optics Express, 2017, 25: 17382–17392. DOI: 10.1364/OE.25.017382
|
| [17] |
Ju L B, Zhou C T, Jiang K, et al. Manipulating the topological structure of ultrarelativistic electron beams using Laguerre-Gaussian laser pulse. New Journal of Physics, 2018, 20: 063004. DOI: 10.1088/1367-2630/aac68a
|
| [18] |
Zhu X L, Chen M, Weng S M, et al. Single-cycle terawatt twisted-light pulses at midinfrared wavelengths above 10 µm. Physical Review Applied, 2019, 12: 054024. DOI: 10.1103/PhysRevApplied.12.054024
|
| [19] |
Tikhonchuk V T, Korneev P, Dmitriev E, et al. Numerical study of momentum and energy transfer in the interaction of a laser pulse carrying orbital angular momentum with electrons. High Energy Density Physics, 2020, 37: 100863. DOI: 10.1016/j.hedp.2020.100863
|
| [20] |
Nuter R, Korneev P, Thiele I, et al. Plasma solenoid driven by a laser beam carrying an orbital angular momentum. Physical Review E, 2018, 98: 033211. DOI: 10.1103/PhysRevE.98.033211
|
| [21] |
Blackman D R, Nuter R, Korneev P, et al. Nonlinear Landau damping of plasma waves with orbital angular momentum. Physical Review E, 2020, 102: 033208. DOI: 10.1103/PhysRevE.102.033208
|
| [22] |
Longman A, Fedosejevs R. Kilo-Tesla axial magnetic field generation with high intensity spin and orbital angular momentum beams. Physical Review Research, 2021, 3: 043180. DOI: 10.1103/PhysRevResearch.3.043180
|
| [23] |
Leblanc A, Denoeud A, Chopineau L, et al. Plasma holograms for ultrahigh-intensity optics. Nature Physics, 2017, 13: 440–443. DOI: 10.1038/nphys4007
|
| [24] |
Denoeud A, Chopineau L, Leblanc A, et al. Interaction of ultraintense laser vortices with plasma mirrors. Physical Review Letters, 2017, 118: 033902. DOI: 10.1103/PhysRevLett.118.033902
|
| [25] |
Longman A, Salgado C, Zeraouli G, et al. Off-axis spiral phase mirrors for generating high-intensity optical vortices. Optics Letters, 2020, 45: 2187–2190. DOI: 10.1364/OL.387363
|
| [26] |
Bae J Y, Jeon C, Pae K H, et al. Generation of low-order Laguerre-Gaussian beams using hybrid-machined reflective spiral phase plates for intense laser-plasma interactions. Results in Physics, 2020, 19: 103499. DOI: 10.1016/j.rinp.2020.103499
|
| [27] |
Aboushelbaya R, Glize K, Savin A F, et al. Measuring the orbital angular momentum of high-power laser pulses. Physics of Plasmas, 2020, 27: 053107. DOI: 10.1063/5.0005140
|
| [28] |
Zeng X, Zheng S, Cai Y, et al. Generation and imaging of a tunable ultrafast intensity-rotating optical field with a cycle down to femtosecond region. High Power Laser Science and Engineering, 2020, 8: e3. DOI: 10.1017/hpl.2020.1
|
| [29] |
Shi Y, Vieira J, Trines R M G M, et al. Magnetic field generation in plasma waves driven by copropagating intense twisted lasers. Physical Review Letters, 2018, 121: 145002. DOI: 10.1103/PhysRevLett.121.145002
|
| [30] |
Blackman D R, Nuter R, Korneev P, et al. Kinetic plasma waves carrying orbital angular momentum. Physical Review E, 2019, 100: 013204. DOI: 10.1103/PhysRevE.100.013204
|
| [31] |
Blackman D R, Nuter R, Korneev P, et al. Twisted kinetic plasma waves. Journal of Russian Laser Research, 2019, 40: 419–428. DOI: 10.1007/s10946-019-09822-3
|
| [32] |
Arber T D, Bennett K, Brady C S, et al. Contemporary particle-in-cell approach to laser-plasma modelling. Plasma Physics and Controlled Fusion, 2015, 57: 113001. DOI: 10.1088/0741-3335/57/11/113001
|
| [33] |
Fedele R, de Angelis U, Katsouleas T. Generation of radial fields in the beat-wave accelerator for Gaussian pump profiles. Physical Review A, General Physics, 1986, 33: 4412–4414. DOI: 10.1103/PhysRevA.33.4412
|
| [34] |
Gorbunov L, Mora P, Antonsen T M Jr. Magnetic field of a plasma wake driven by a laser pulse. Physical Review Letters, 1996, 76: 2495–2498. DOI: 10.1103/PhysRevLett.76.2495
|
| [35] |
Gorbunov L M, Mora P, Antonsen T M. Quasistatic magnetic field generated by a short laser pulse in an underdense plasma. Physics of Plasmas, 1997, 4: 4358–4368. DOI: 10.1063/1.872598
|
| [36] |
Dawson J M. Nonlinear electron oscillations in a cold plasma. Physical Review, 1959, 113: 383–387. DOI: 10.1103/PhysRev.113.383
|
| [37] |
Cowley J, Thornton C, Arran C, et al. Excitation and control of plasma wakefields by multiple laser pulses. Physical Review Letters, 2017, 119: 044802. DOI: 10.1103/PhysRevLett.119.044802
|
| [38] |
EPOCH Particle-In-Cell code for plasma simulations. https://github.com/epochpic/epochpic.github.io. Accessed April 10, 2022.
|
| Laser parameters | LG-CP beam1 | LG-CP beam 2 |
| Electric field amplitude ( E_{pl} ) | E_{1(y,z)} = 1.0 TV/m | E_{2(y,z)} = 1.0 TV/m |
| Wavelength | \lambda_1 = 0.8\; μm | \lambda_2 = 0.89 \; μm |
| Twist index | l_1 = −1 | l_2 = 1 |
| Pulse duration (Gaussian shape) | \tau_{\rm {g}} = 200 fs (75 cycles) | \tau_{\rm {g}} = 200 fs (67.4 cycles) |
| Focal spot size (1/{e} electric field) | w_{\rm{b}} = 10 μm | w_{\rm{b}} = 10 μm |
| Laser propagation direction | +x | +x |
| Other parameters | ||
| Electron density | n_{\rm{e}} = 0.01\;n_{ {\rm{c} } } | |
| Simulation box ( x\times y \times z ) | 30 {\rm{\mu }}m × \; 50 \; \mum × \; 50 \;\mum | |
| Cell number ( x\times y \times z ) | 600 × 800 × 800 | |
| Macroparticles per cell for each species | 2 | |