
Sheared E × B flow has been frequently observed to excite instability in space plasma. In this study, two methods – the interpenetrating plasma and ring electrode methods – were developed in the Keda Space Plasma EXperiment (KSPEX) device to trigger sheared E × B flow. Both methods produce sheared E × B flow by generating a radial electric field. The results of the experiment indicated that plasma instabilities in the ion cyclotron range can be excited by these methods. Therefore, the methods reported here are important for research on the mechanism for generating sheared flow-driven plasma instabilities, which may enrich our understanding of geospace physics.
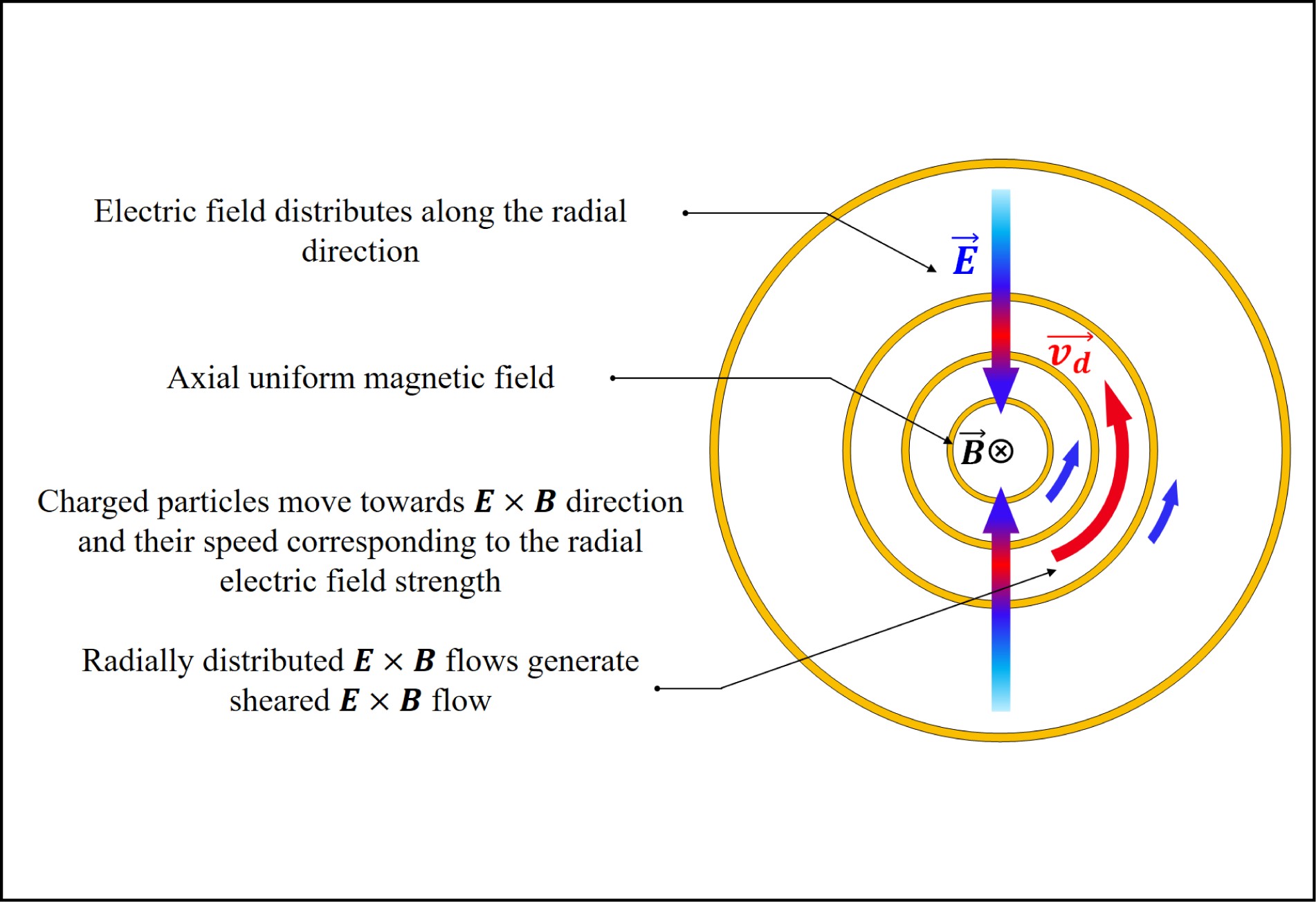
With the electric field varying along the radial position, the corresponding E×B flow in different strengths yield sheared E×B flow.
Figure
5.
(a) Radial profiles of the plasma potential and the electric field as the outer rings biased at 10 V and the inner ring biased at
Figure
7.
PSD of potential fluctuation versus different biasing voltages on the ring electrodes. The legend is written in the format of “bias on the outer ring / bias on the inner ring” while “
| [1] |
Makarevich R A, Crowley G, Azeem I, et al. Auroral E-region as a source region for ionospheric scintillation. Journal of Geophysical Research:Space Physics, 2021, 126 (5): e2021JA029212. DOI: 10.1029/2021JA029212
|
| [2] |
Hysell D L. From instability to irregularities. In: The Dynamical Ionosphere. Amsterdam, Netherlands: Elsevier, 2020: 137-167.
|
| [3] |
Fejer B G, Kelley M C. Ionospheric irregularities. Reviews of Geophysics, 1980, 18 (2): 401–454. DOI: 10.1029/RG018i002p00401
|
| [4] |
Wernik A W, Secan J A, Fremouw E J. Ionospheric irregularities and scintillation. Advances in Space Research, 2003, 31 (4): 971–981. DOI: 10.1016/S0273-1177(02)00795-0
|
| [5] |
Balsley B B, Ecklund W L. VHF power spectra of the radar aurora. Journal of Geophysical Research, 1972, 77 (25): 4746–4760. DOI: 10.1029/JA077i025p04746
|
| [6] |
Kintner P, D'Angelo N. A transverse Kelvin-Helmholtz instability in a magnetized plasma. Journal of Geophysical Research, 1977, 82 (10): 1628–1630. DOI: 10.1029/JA082i010p01628
|
| [7] |
Pradhan S M, Tiwari R S, Singh B, et al. Ionospheric irregularities plasma instability. Indian Journal of Radio Space Physics, 1972, 1: 218–220.
|
| [8] |
Liu Y, Shi P, Zhang X, et al. Laboratory plasma devices for space physics investigation. Review of Scientific Instruments, 2021, 92 (7): 071101. DOI: 10.1063/5.0021355
|
| [9] |
Ganguli G, Keskinen M J, Romero H, et al. Coupling of microprocesses and macroprocesses due to velocity shear: An application to the low-altitude ionosphere. Journal of Geophysical Research:Space Physics, 1994, 99 (A5): 8873–8889. DOI: 10.1029/93JA03181
|
| [10] |
Linson L M, Workman J B. Formation of striations in ionospheric plasma clouds. Journal of Geophysical Research, 1970, 75 (16): 3211–3219. DOI: 10.1029/JA075i016p03211
|
| [11] |
Tsuda T, Sato T, Matsushita S. Ionospheric irregularities and the cross-field plasma instability. Journal of Geophysical Research, 1969, 74 (11): 2923–2932. DOI: 10.1029/JA074i011p02923
|
| [12] |
Ganguli G, Lee Y C, Palmadesso P J. Electron–ion hybrid mode due to transverse velocity shear. The Physics of Fluids, 1988, 31 (10): 2753–2756. DOI: 10.1063/1.866982
|
| [13] |
Ganguli G, Lee Y C, Palmadesso P J. Kinetic theory for electrostatic waves due to transverse velocity shears. The Physics of Fluids, 1988, 31 (4): 823–838. DOI: 10.1063/1.866818
|
| [14] |
Nykyri K, Otto A. Plasma transport at the magnetospheric boundary due to reconnection in Kelvin-Helmholtz vortices. Geophysical Research Letters, 2001, 28 (18): 3565–3568. DOI: 10.1029/2001GL013239
|
| [15] |
Johnson J R, Wing S, Delamere P A. Kelvin Helmholtz instability in planetary magnetospheres. Space Science Reviews, 2014, 184 (1-4): 1–31. DOI: 10.1007/s11214-014-0085-z
|
| [16] |
Jassby D L. Transverse velocity shear instabilities within a magnetically confined plasma. The Physics of Fluids, 1972, 15 (9): 1590–1604. DOI: 10.1063/1.1694135
|
| [17] |
Thomas Jr E, Jackson J D, Wallace E A, et al. Observations of low frequency oscillations due to transverse sheared flows. Physics of Plasmas, 2003, 10 (5): 1191–1194. DOI: 10.1063/1.1567287
|
| [18] |
Amatucci W E, Koepke M E, Carroll III J J, et al. Observation of ion-cyclotron turbulence at small values of magnetic-field-aligned current. Geophysical Research Letters, 1994, 21 (15): 1595–1598. DOI: 10.1029/94GL00881
|
| [19] |
Yoshinuma M, Inutake M, Hatakeyama R, et al. Control of radial potential profile and related low-frequency fluctuations in an ECR-produced plasma. Physics Letters A, 1999, 255 (4–6): 301–306. DOI: 10.1016/S0375-9601(99)00171-1
|
| [20] |
D'Angelo N. Kelvin-Helmholtz instability in a fully ionized plasma in a magnetic field. The Physics of Fluids, 1965, 8 (9): 1748–1750. DOI: 10.1063/1.1761496
|
| [21] |
Teodorescu C, Reynolds E W, Koepke M E. Observation of inverse ion-cyclotron damping induced by parallel-velocity shear. Physical Review Letters, 2002, 89 (10): 105001. DOI: 10.1103/PhysRevLett.89.105001
|
| [22] |
Fujita H, Yagura S, Harada T, et al. Observation of potential relaxation instability in a bounded discharge plasma. IEEE Transactions on Plasma Science, 1987, 15 (4): 445–451. DOI: 10.1109/TPS.1987.4316728
|
| [23] |
Kaneko T, Odaka Y, Tada E, et al. Generation and control of field-aligned flow velocity shear in a fully ionized collisionless plasma. Review of Scientific Instruments, 2002, 73 (12): 4218–4222. DOI: 10.1063/1.1518791
|
| [24] |
Liu Y, Zhang Z, Lei J, et al. Design and construction of Keda Space Plasma Experiment (KSPEX) for the investigation of the boundary layer processes of ionospheric depletions. Review of Scientific Instruments, 2016, 87 (9): 093504. DOI: 10.1063/1.4962406
|
| [25] |
Kaneko T, Tsunoyama H, Hatakeyama R. Drift-wave instability excited by field-aligned ion flow velocity shear in the absence of electron current. Physical Review Letters, 2003, 90 (12): 125001. DOI: 10.1103/PhysRevLett.90.125001
|
| [26] |
Liu Y, Lei J, Yu P, et al. Laboratory generation of broadband ELF waves by inhomogeneous plasma flow. Geophysical Research Letters, 2017, 44 (4): 1634–1640. DOI: 10.1002/2016GL072232
|
| [27] |
D'Angelo N, Pécseli H L, Petersen P I. The Farley instability: A laboratory test. Journal of Geophysical Research, 1974, 79 (31): 4747–4751. DOI: 10.1029/JA079i031p04747
|
| [28] |
Desjardins T R, Gilmore M. Dynamics of flows, fluctuations, and global instability under electrode biasing in a linear plasma device. Physics of Plasmas, 2016, 23 (5): 055710. DOI: 10.1063/1.4948282
|
| Variable | Description | |
| Ln_helpfulness | The logarithmic form of helpfulness votes received by the review j of hotel i | |
| Reviewer_impact | Expertiseijp | The reviewer expertise p of review j of hotel i, including Newcomer, Master, Expert |
| Experienceijq | The reviewer experience q of review j of hotel i, including Sum_reviews (the sum of reviews s/he posted), Avg_pics (the average number of pictures s/he posted per review) | |
| Avg_helpsij | The average number of helpfulness votes reviewer gained per review of hotel i | |
| Trip_purposeijm | If the trip purpose of review j of hotel i is m, its value equals 1; otherwise 0. Specifically, m refers to Family, Friends, Business, Couple, Solo, Other, ResforO (reserving for other people) | |
| Review_informativeness |
Lengthij | The number of words in the review j of hotel i |
| UGPsij | The number of use-generated photos in the review j of hotel i | |
| Timelinessij | The interval between the review time of the review j of the hotel i and its check-in time | |
| Hotel_MRs | Replyij | If the review j of hotel i is replied to by the hotel manager, the value equals 1 |
| Reply_lengthij | The number of reply words in the review j of hotel i | |
| Controlvariables | HSCai | The star/diamond level of hotel i |
| Priceij | The room price of review j of hotel i (take log) | |
| Ratingij | The rating of the review j of hotel i | |
| Review_ageij | The time elapsed (in days) since the date on which review j of hotel i was posted | |
| a: Hotels on Ctrip.com are classified as either star-hotels or diamond-hotels. If a hotel is rated by the Ministry of Culture and Tourism of the People’s Republic of China, its star level is shown on its web page; otherwise, the diamond-level determined by Ctrip is shown on the web page. | ||
| Variable | Min | Max | Mean | SD | |
| Expertise | Newcomer | 0 | 1 | ||
| Master | 0 | 1 | |||
| Expert | 0 | 1 | |||
| Experience | Sum_reviews | 0 | |||
| Avg_pics | 0 | 9 | 0.316 | 0.814 | |
| Reviewer historical performance | Avg_helps | 0 | 150.091 | 0.241 | |
| Trip_purpose | Family | 0 | 1 | ||
| Friends | 0 | 1 | |||
| Business | 0 | 1 | |||
| Couple | 0 | 1 | |||
| Solo | 0 | 1 | |||
| Other | 0 | 1 | |||
| ResforO | 0 | 1 | |||
| Review_informativeness | Length | 0 | |||
| UGPs | 0 | 9 | |||
| Timeliness | 0 | 394 | |||
| Hotel_MRs | Reply | 0 | 1 | ||
| Reply_length | 49 | 129.33 | |||
| Control variables | HSC | 2 | 5 | 3.908 | 0.94 |
| Price | 6.101 | ||||
| Rating | 1 | 5 | |||
| Review_age | 0 | ||||
| Dependent variables | Ln_helpfulness | 0 | |||
| The sample size of Reply_length is |
|||||
| Review helpfulness | Frequency | Percent |
| 0 | 100731 | 91.67% |
| 1 | 5636 | 5.13% |
| 2 | 1426 | 1.30% |
| 3–10 | 1.56% | |
| >11 | 375 | 0.33% |
| Total | 109884 | 100% |
| Model 1 | Model 2 | Model 3 | Model4 | ||||||||
| Ln_helpfulness | Review_informativeness | Hotel_MRs | Ln_helpfulness | ||||||||
| Length | UGPs | Timeliness | Reply | Reply_length | |||||||
| Expertise | Master | −0.109*** | −0.496* | −0.035*** | 15.587*** | 0.073*** | 2.923*** | −0.036* | |||
| (0.02) | (0.29) | (0.01) | (0.45) | (0.01) | (0.54) | (0.02) | |||||
| Expert | −0.152*** | 6.821*** | −0.003 | 13.430*** | 0.186*** | 7.505*** | −0.140*** | ||||
| (0.03) | (0.40) | (0.01) | (0.66) | (0.02) | (0.73) | (0.03) | |||||
| Experience | Sum_reviews | 0.025** | 3.497*** | 0.002** | 0.351 | −0.026*** | −1.161*** | 0.004 | |||
| (0.01) | (0.20) | (0.00) | (0.28) | (0.01) | (0.24) | (0.01) | |||||
| Avg_pics | 0.410*** | 12.998*** | 0.865*** | −3.790*** | 0.014 | 1.007** | 0.011 | ||||
| (0.01) | (0.31) | (0.01) | (0.20) | (0.01) | (0.41) | (0.01) | |||||
| Avg_helps | 0.123*** | 1.064*** | 0.004** | −0.681*** | −0.071 *** | −1.130*** | 0.106*** | ||||
| (0.00) | (0.17) | (0.00) | (0.13) | (0.01) | (0.20) | (0.00) | |||||
| Trip_purpose | Family | 0.475*** | 10.418*** | 0.146*** | −8.180*** | 0.161*** | 12.302*** | 0.194*** | |||
| (0.06) | (0.59) | (0.01) | (1.28) | (0.04) | (1.27) | (0.06) | |||||
| Friend | 0.337*** | 5.999*** | 0.104*** | −4.937*** | 0.017 | 4.454*** | 0.152*** | ||||
| (0.06) | (0.60) | (0.01) | (1.31) | (0.04) | (1.28) | (0.06) | |||||
| Business | 0.228*** | 1.080** | 0.102*** | −5.459*** | −0.041 | 2.112* | 0.097* | ||||
| (0.06) | (0.50) | (0.01) | (1.19) | (0.03) | (1.12) | (0.05) | |||||
| Couple | 0.393*** | 6.711*** | 0.125*** | −6.918*** | 0.004 | 2.307* | 0.161*** | ||||
| (0.06) | (0.69) | (0.01) | (1.33) | (0.04) | (1.33) | (0.06) | |||||
| Solo | 0.359*** | 6.309*** | 0.153*** | −7.029*** | −0.042 | 10.910*** | 0.132** | ||||
| (0.07) | (0.71) | (0.01) | (1.36) | (0.04) | (1.51) | (0.06) | |||||
| Other | 0.508*** | 7.182*** | 0.120*** | −11.141*** | 0.009 | 1.104 | 0.244*** | ||||
| (0.07) | (0.75) | (0.01) | (1.41) | (0.04) | (1.47) | (0.06) | |||||
| Review_informativeness | Length | 0.004*** | 0.192*** | 0.007*** | |||||||
| (0.00) | (0.01) | (0.00) | |||||||||
| UGPs | 0.029*** | 1.070*** | 0.255*** | ||||||||
| (0.01) | (0.30) | (0.01) | |||||||||
| Timeliness | −0.001*** | −0.024*** | −0.009*** | ||||||||
| (0.00) | (0.00) | (0.00) | |||||||||
| Hotel_MRs | Reply | 0.073* | |||||||||
| (0.04) | |||||||||||
| Reply_length | −0.001*** | ||||||||||
| (0.00) | |||||||||||
| Control variables included & hotel heterogeneity effect included | |||||||||||
| Constant | −1.111 | 103.179*** | −0.467*** | 28.026*** | 45.861*** | 0.965 | −1.229 | ||||
| (3.87) | (14.33) | (0.07) | (10.81) | (10.30) | (4.02) | (3.58) | |||||
| N | |||||||||||
| R2 | 0.161 | 0.148 | 0.451 | 0.052 | 0.602 | 0.231 | |||||
| ***significance: p < 0.01, **significance: p < 0.05, *significance: p < 0.1. | |||||||||||
| Reviewer_impact ⇒ Review_informativeness⇒ Ln_helpfulness |
Reviewer_impact and Trip_purpose | Conf. | [95% conf. interval] | |
| Reviewer_impact⇒ Length⇒ Ln_helpfulness |
Master | − |
− |
− |
| Expert | ||||
| Sum_reviews | ||||
| Avg_pics | ||||
| Avg_helps | ||||
| Family | ||||
| Friends | ||||
| Business | ||||
| Couple | ||||
| Solo | ||||
| Others | ||||
| Reviewer_impact⇒ UGPs⇒ Ln_helpfulness |
Master | − |
− |
− |
| Expert | − |
− |
− |
|
| Sum_reviews | − |
− |
− |
|
| Avg_pics | ||||
| Avg_helps | ||||
| Family | ||||
| Friends | ||||
| Business | ||||
| Couple | ||||
| Solo | ||||
| Others | ||||
| Reviewer_impact⇒ Timeliness⇒ Ln_helpfulness |
Master | − |
− |
− |
| Expert | − |
− |
− |
|
| Sum_reviews | − |
− |
− |
|
| Avg_pics | ||||
| Avg_helps | ||||
| Family | ||||
| Friends | ||||
| Business | ||||
| Couple | ||||
| Solo | ||||
| Others | ||||
| ***significance: p < 0.01, **significance: p < 0.05, *significance: p < 0.1. | ||||
| Reviewer_impact⇒ Hotel_MRs ⇒Ln_helpfulness |
Reviewer_impact | Conf. | [95% conf. interval] | |
| Reviewer_impact⇒ Reply⇒ Ln_helpfulness |
Master | − |
− |
− |
| Expert | − |
− |
− |
|
| Sum_reviews | −2.70E–05 | − |
||
| Avg_pics | − |
− |
− |
|
| Avg_helps | ||||
| Family | − |
− |
− |
|
| Friends | − |
|||
| Business | − |
|||
| Couple | − |
|||
| Solo | − |
|||
| Others | − |
− |
||
| Reviewer_impact⇒ Reply_length⇒ Ln_helpfulness |
Master | − |
||
| Expert | ||||
| Sum_reviews | −3.46E–06 | −7.67E–06 | 7.51E–07 | |
| Avg_pics | 4.97E–06 | −7.41E–06 | ||
| Avg_helps | −1.31E−05 | − |
4.76E–06 | |
| Family | ||||
| Friends | − |
|||
| Business | − |
|||
| Couple | − |
− |
||
| Solo | − |
|||
| Others | − |
|||
| ***significance: p < 0.01, **significance: p < 0.05, *significance: p < 0.1. | ||||
| Reviewer_impact⇒ Review_informativeness ⇒ Hotel_MRs ⇒ Ln_helpfulness |
Reviewer_impact | Conf. | [95% conf. interval] | |
| Reviewer_impact⇒ Length⇒ Reply⇒ Ln_helpfulness |
Master | − |
− |
|
| Expert | − |
− |
− |
|
| Sum_reviews | −3.00E–05*** | − |
− |
|
| Avg_pics | − |
− |
− |
|
| Avg_helps | − |
− |
− |
|
| Family | − |
− |
− |
|
| Friends | − |
− |
− |
|
| Business | − |
− |
− |
|
| Couple | − |
− |
− |
|
| Solo | − |
− |
− |
|
| Others | − |
− |
− |
|
| Reviewer_impact⇒ Pictures⇒ Reply⇒ Ln_helpfulness |
Master | |||
| Expert | − |
|||
| Sum_reviews | 2.15E–06** | 5.01E–07 | 3.80E–06 | |
| Avg_pics | − |
− |
− |
|
| Avg_helps | −7.05E–06** | − |
−3.78E–07 | |
| Family | − |
− |
− |
|
| Friends | − |
− |
− |
|
| Business | − |
− |
− |
|
| Couple | − |
− |
− |
|
| Solo | − |
− |
− |
|
| Others | − |
− |
− |
|
| Reviewer_impact⇒ Timeliness⇒ Reply⇒ Ln_helpfulness |
Master | |||
| Expert | ||||
| Sum_reviews | 1.58E–06** | 3.64E–07 | 2.80E–06 | |
| Avg_pics | −1.30E-05*** | − |
−4.93E–06 | |
| Avg_helps | −9.07E–06*** | − |
−2.44E–06 | |
| Family | − |
− |
− |
|
| Friends | − |
− |
− |
|
| Business | − |
− |
− |
|
| Couple | − |
− |
− |
|
| Solo | − |
− |
− |
|
| Others | − |
− |
− |
|
| Reviewer_impact⇒ Length⇒ Reply_length⇒ Ln_helpfulness |
Master | − |
− |
|
| Expert | 5.54E–06 | |||
| Sum_reviews | 1.80E–06** | 8.94E–09 | 3.60E–06 | |
| Avg_pics | −1.17E–07** | 2.85E–07 | ||
| Avg_helps | 6.09E–06** | 2.40E–08 | ||
| Family | ||||
| Friends | 1.31E–06 | |||
| Business | − |
|||
| Couple | ||||
| Solo | ||||
| Others | ||||
| Reviewer_impact⇒ UGPs⇒ Reply_length⇒ Ln_helpfulness |
Master | −2.02E–05 | − |
4.19E–06 |
| Expert | − |
− |
||
| Sum_reviews | −1.17E–07 | −2.62E–07 | −2.82E–07 | |
| Avg_pics | 4.37E–06 | −9.32E–07 | 9.66E–06 | |
| Avg_helps | 3.83E–07 | −1.51E–07 | 9.17E–07 | |
| Family | − |
|||
| Friends | − |
|||
| Business | 3.36E–05 | −8.14E–06 | ||
| Couple | − |
|||
| Solo | − |
|||
| Others | − |
|||
| Reviewer_impact⇒ Timeliness⇒ Reply_length⇒ Ln_helpfulness |
Master | − |
− |
5.93E–06 |
| Expert | − |
− |
4.26E–06 | |
| Sum_reviews | −1.17E–07* | −2.56E–07 | 2.18E–08 | |
| Avg_pics | 9.66E–07* | −4.04E–08 | 1.97E–06 | |
| Avg_helps | 6.71E–07* | −7.20E–08 | 1.41E–06 | |
| Family | −5.26E–06 | |||
| Friends | −9.76E–06 | |||
| Business | −7.05E–06 | |||
| Couple | −7.01E–06 | |||
| Solo | −4.42E–06 | |||
| Others | −6.93E–06 | |||
| ***significance: p < 0.01, **significance: p < 0.05, *significance: p < 0.1. | ||||
| (1) | (2) | (3) | (4) | |
| HSC = 2 | HSC = 3 | HSC = 4 | HSC = 5 | |
| Reply_length | 0.001 | 0.000 | −0.000 | −0.004*** |
| (0.00) | (0.00) | (0.00) | (0.00) | |
| Reply | −0.256** | −0.391*** | 0.320*** | −0.005 |
| (0.12) | (0.10) | (0.05) | (0.08) | |
| Length | 0.007*** | 0.009*** | 0.006*** | 0.007*** |
| (0.00) | (0.00) | (0.00) | (0.00) | |
| UGPs | 0.138*** | 0.212*** | 0.292*** | 0.236*** |
| (0.03) | (0.02) | (0.01) | (0.01) | |
| Timeliness | −0.006*** | −0.008*** | −0.008*** | −0.010*** |
| (0.00) | (0.00) | (0.00) | (0.00) | |
| Master | −0.103* | 0.031 | 0.013 | −0.022 |
| (0.06) | (0.05) | (0.03) | (0.04) | |
| Expert | −0.155 | −0.043 | −0.158*** | −0.088** |
| (0.10) | (0.09) | (0.05) | (0.04) | |
| Sum_reviews | 0.005 | −0.020 | −0.029 | 0.007 |
| (0.02) | (0.05) | (0.04) | (0.01) | |
| Avg_pics | 0.072** | −0.009 | 0.021 | −0.039** |
| (0.03) | (0.03) | (0.02) | (0.02) | |
| Avg_helps | 0.073*** | 0.046*** | 0.109*** | 0.115*** |
| (0.01) | (0.01) | (0.01) | (0.00) | |
| Family | 0.572*** | −0.032 | 0.195** | 0.097 |
| (0.22) | (0.16) | (0.09) | (0.08) | |
| Friend | 0.563** | −0.147 | 0.099 | 0.181** |
| (0.22) | (0.16) | (0.10) | (0.09) | |
| Business | 0.509** | −0.123 | 0.051 | 0.065 |
| (0.21) | (0.15) | (0.09) | (0.08) | |
| Couple | 0.532** | 0.023 | 0.142 | 0.084 |
| (0.23) | (0.16) | (0.10) | (0.09) | |
| Solo | 0.537** | −0.080 | 0.157 | 0.106 |
| (0.22) | (0.17) | (0.10) | (0.09) | |
| Others | 0.578*** | 0.052 | 0.205** | 0.232** |
| (0.22) | (0.17) | (0.10) | (0.09) | |
| Ln_price | 0.005 | −0.116 | −0.421*** | −0.077 |
| (0.11) | (0.16) | (0.05) | (0.08) | |
| Rating | −0.255*** | −0.383*** | −0.431*** | −0.203*** |
| (0.03) | (0.03) | (0.02) | (0.02) | |
| Duration | 0.001*** | 0.001*** | 0.001*** | −0.002*** |
| (0.00) | (0.00) | (0.00) | (0.00) | |
| Hotel effect included | ||||
| _cons | −3.197*** | −0.394 | 1.671*** | −0.546 |
| (0.66) | (0.87) | (0.40) | (0.50) | |
| N | ||||
| R2 | 0.247 | 0.237 | 0.254 | 0.247 |
| ***significance: p < 0.01, **significance: p < 0.05, *significance: p < 0.1. | ||||