ISSN 0253-2778
CN 34-1054/N
ISSN 0253-2778
CN 34-1054/N
School of Mathematics and Statistics, Nanjing University of Science and Technology, Nanjing 210094, China
Zhenghan Shen is currently a postdoctoral fellow at Nanjing University of Science and Technology. He received his PhD degree in Geometric Analysis under the tutelage of Prof. Xi Zhang from University of Science and Technology of China. His research interests focus on the Higgs bundle, Hermitian-Einstein metric and Hermitian-Yang-Mills flow
Zhenghan Shen, E-mail: mathszh@njust.edu.cn
The curvature estimate of the Yang-Mills-Higgs flow on Higgs bundles over compact Kähler manifolds is studied. Under the assumptions that the Higgs bundle is non-semistable and the Harder-Narasimhan-Seshadri filtration has no singularities with length one, it is proved that the curvature of the evolved Hermitian metric is uniformly bounded.
Using the decomposition of Donaldson’s functional and the properties of Harder-Nasimhan-Seshadri filtration, we get the C0-bound of the rescaled metrics
The curvature estimate of the Yang-Mills-Higgs flow on Higgs bundles over compact Kähler manifolds is studied. Under the assumptions that the Higgs bundle is non-semistable and the Harder-Narasimhan-Seshadri filtration has no singularities with length one, it is proved that the curvature of the evolved Hermitian metric is uniformly bounded.
Let 

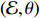


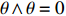
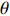

|
μω(F)=degω(F)rank(F)<(⩽)μω(E)=degω(E)rank(E)
|
where 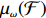
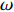
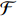
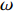

|
degω(F)=∫Mc1(F)∧ωn−1(n−1)!,
|
where 
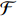
Let Σε be a set of singularities, where 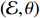
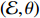
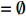
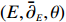
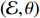
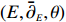
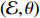

Given an unstable torsion-free coherent Higgs sheaf 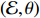
|
0=E0⊂E1⊂⋯⊂Ek=E
|
such that the quotient 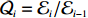
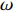

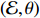
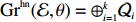
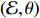
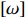
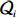
|
0=Ei,0⊂Ei,1⊂⋯⊂Ei,ki=Qi
|
such that the quotients 
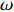
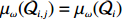
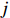
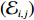
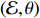
|
Grhns(E,θ)=k⨁i=1ki⨁j=1Qi,j
|
is uniquely determined by the isomorphism class of 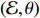
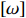
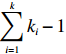
Given a Hermitian metric 
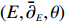
|
ˉ∂θ:=ˉ∂E+θ,D′:=∂H+θ∗H,DH,θ=ˉ∂+∂H
|
where 
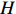

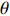
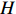
|
FH,θ=FH+[θ,θ∗H]+∂Hθ+ˉ∂Eθ∗H
|
where 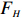

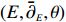
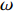
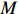
|
√−1Λω(FH+[θ,θ∗])=λE,ωIdE
|
where 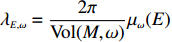

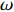
Let 
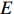
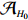


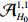
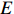

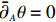
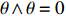
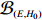
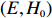
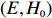

|
∂A∂t=−D∗AFA−√−1(∂AΛω−ˉ∂AΛω)[θ,θ∗],∂θ∂t=−[√−1Λω(FA+[θ,θ∗]),θ]}
|
The Yang-Mills flow was first introduced by Atiyah-Bott in Ref. [13]. Simpson[2] induces the following Hermitian-Yang-Mills-Higgs (HYMH) flow for Hermitian metrics 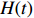
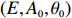
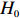
|
H−1∂H∂t=−2(√−1Λω(FH+[θ0,θ∗H0])−λE⋅IdE),H(0)=H0}
|
Simpson[2] proved the long-time existence of the Hermitian-Yang-Mills-Higgs flow and demonstrated convergence under the condition that the Higgs bundle is stable. In Ref. [14], Li and Zhang showed that by choosing complex gauge transformations 
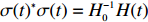
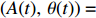

According to Uhlenbeck's compactness[15,16], for any sequence 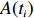

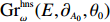
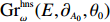


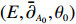
Theorem 1.1. Let 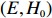
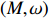

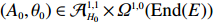

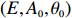

|
sup
|
If the algebraic singular set Σalg 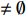
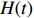
|
\tag{11} \begin{equation} |F_{H(t)}+[\theta_0,\theta_0^{*H}]+\bar{\partial}_E\theta_0^{*H}+\partial_H\theta_0|_H^2 = |F_A+[\theta,\theta^*]|_{H_0}^2+2|\partial_A\theta|_{H_0}^2 \end{equation}
|
Therefore, we only need to estimate the curvature tensor 
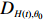
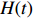
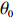
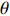
|
\tag{12} \begin{equation} 0\rightarrow (S,\bar{\partial}_S,\theta_S)\rightarrow (E,\bar{\partial}_E,\theta)\rightarrow (Q,\bar{\partial}_Q,\theta_Q)\rightarrow 0 \end{equation}
|
such that 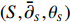
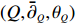
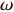
|
\tag{13} \begin{equation} \mu_{\omega}(S)>\mu_{\omega}(E)>\mu_{\omega}(Q) \end{equation}
|
Let 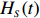


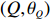
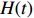

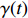

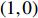
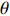
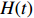
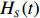
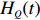
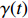
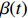
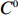

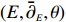

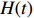
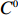
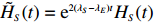
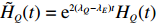
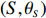
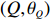
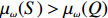

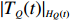
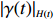
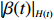
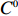
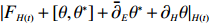
The remainder of this paper is organized as follows. In Section 2, we derive the evolution equations for the induced metrics 


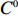
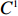
Let 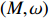


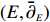
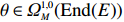
|
\begin{equation*} \bar{\partial}_{E}\theta = 0 \quad \rm {and} \quad \theta\wedge \theta = 0. \end{equation*}
|
Let 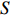
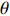
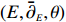
|
\tag{14} \begin{equation} 0\rightarrow (S,\bar{\partial}_S,\theta_S) \rightarrow (E,\bar{\partial}_E,\theta) \rightarrow (Q,\bar{\partial}_Q,\theta_Q)\rightarrow 0 \end{equation}
|
where 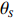

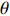
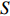
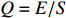
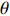
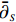
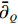
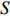

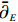
Given a Hermitian metric 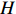
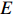
|
\tag{15} \begin{equation} f_H: S\oplus Q \rightarrow E,\quad (X,[Y])\mapsto i(X)+({\rm Id}_E-\pi_H)(Y) \end{equation}
|
where 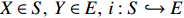

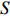
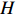
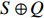
|
\tag{16} \begin{equation} f^*_{H}(H) = \begin{pmatrix} H_S&0\\ 0&H_Q \end{pmatrix},\quad f_{H}^{*}(\bar{\partial}_E) = \begin{pmatrix} \bar{\partial}_S&\gamma\\ 0&\bar{\partial}_Q \end{pmatrix}, \quad f^*_H(\theta) = \begin{pmatrix} \theta_S&\beta\\ 0&\theta_Q \end{pmatrix} \end{equation}
|
where 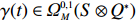
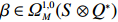
|
\tag{17} \begin{equation} \begin{split} \quad f^*_H(F_{H,\theta}) = &f^*_H(F_H+[\theta,\theta^{*H}]+\partial_H\theta+\bar{\partial}_E\theta^{*H}) = \\& \begin{pmatrix} F_{H_S}-\gamma\wedge \gamma^{*H}&\partial_{S\otimes Q^*}\gamma\\ -\bar{\partial}_{S^*\otimes Q}\gamma^{*H}&F_{H_Q}-\gamma^{*H}\wedge \gamma \end{pmatrix} +\\&\begin{pmatrix} \partial_{H_S}\theta_S+\bar{\partial}_S\theta_S^{*H_S}+\gamma\wedge\beta^{*H}-\beta\wedge\gamma^{*H}& \partial_{S\otimes Q^*}\beta+\theta_S^{*H_S}\wedge \gamma+\gamma\wedge \theta_Q^{*H_{Q}}\\ \bar{\partial}_{S^{*}\otimes Q }\beta^{*H}-\gamma^{*H}\wedge \theta_S-\theta_Q\wedge \gamma^{*H}&\partial_{H_Q}\theta_Q+\bar{\partial}_Q\theta_Q^{*H}-\gamma^{*H}\wedge\beta+\beta^{*H}\wedge \gamma \end{pmatrix} +\\ &\begin{pmatrix} [\theta_S,\theta_S^{*H_S}]+\beta\wedge \beta^{*H}&\theta_S^{*H_S}\wedge\beta+\beta\wedge \theta_Q^{*H_Q}\\ \beta^{*H}\wedge \theta_S+\theta_Q\wedge\beta^{*H}&[\theta_Q,\theta_Q^{*H_Q}]+\beta^{*H}\wedge \beta \end{pmatrix} \end{split} \end{equation}
|
Let 
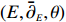
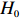
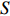
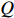
|
\tag{18} \begin{equation} f_{H}^{-1}\frac{\partial f_{H(t)}}{\partial t} = \begin{pmatrix} 0&\varPsi(t)\\ 0&0 \end{pmatrix} \end{equation}
|
and
|
\tag{19} \begin{equation} f_H^*\left(H^{-1}\frac{\partial H}{\partial t}\right) = \begin{pmatrix} H_S^{-1}\dfrac{\partial H_S}{\partial t}&0\\ 0&H_Q^{-1}\dfrac{\partial H_Q}{\partial t} \end{pmatrix}-f_{H}^{-1}\dfrac{\partial f_H}{\partial t}-\left(f_{H}^{-1}\dfrac{\partial f_H}{\partial t}\right)^{*f_{H}^*(H)} \end{equation}
|
Using the flow (9) and Gauss-Codazzi (17) equations, we have
|
\tag{20} \begin{equation} H_S^{-1}\dfrac{\partial H_S}{\partial t} = -2\left(\sqrt{-1}\varLambda_{\omega}(F_{H_S}+[\theta_S,\theta_S^{*H_S}]-\gamma \wedge \gamma^{*H}+\beta\wedge \beta^{*H})-\lambda_E \cdot {\rm Id}_S\right) \end{equation}
|
|
\tag{21} \begin{equation} H_Q^{-1}\frac{\partial H_Q}{\partial t} = -2\left(\sqrt{-1}\varLambda_{\omega}(F_{H_Q}+[\theta_Q,\theta_Q^{*H_Q}]-\gamma^{*H}\wedge\gamma+\beta^{*H}\wedge \beta)-\lambda_E \cdot {\rm Id}_Q\right) \end{equation}
|
|
\tag{22} \begin{equation} f_{H}^{-1}\frac{\partial f_H}{\partial t} = \begin{pmatrix} 0&2\sqrt{-1}\varLambda_{\omega}(\partial_{S\otimes Q^{*}}\gamma+\theta_S^{*H_S}\wedge\beta+\beta\wedge \theta_Q^{*H_Q})\\ 0&0 \end{pmatrix} \end{equation}
|
Now, we consider the evolution of the second fundamental forms 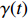

Lemma 2.1. Let 
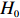
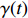
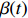
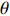
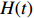
|
\tag{23} \begin{equation} \frac{\partial \gamma(t) }{\partial t} = 2\bar{\partial}_{S\otimes Q^{*}}\left(\sqrt{-1}\varLambda_{\omega}(\partial_{S\otimes Q^{*}}\gamma+\theta_S^{*H_S}\wedge \beta+\beta\wedge \theta_Q^{*H_Q})\right) \end{equation}
|
and
|
\tag{24} \begin{equation} \begin{split} \frac{\partial \beta(t)}{\partial t} = &2\theta_S\circ\left(\sqrt{-1}\Lambda_{\omega}(\partial_{S\otimes Q^{*}}\gamma+\theta_S^{*H_S}\wedge \beta+\beta\wedge \theta_Q^{*H_Q})\right)-\\ \quad &2\left(\sqrt{-1}\Lambda_{\omega}(\partial_{S\otimes Q^{*}}\gamma+\theta_S^{*H_S}\wedge \beta+\beta\wedge \theta_Q^{*H_Q})\right)\circ \theta_Q \end{split} \end{equation}
|
Proof. For simplicity, we let 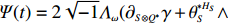
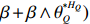
|
\tag{25} \begin{equation} \bar{\partial}f_H = \bar{\partial}_E\circ f_H-f_{H}\circ \bar{\partial}_{S\otimes Q} \end{equation}
|
and then
|
\tag{26} \begin{equation} f_H^{-1}\bar{\partial}f_H = f_{H}^{*}(\bar{\partial}_E)-\bar{\partial}_{S\oplus Q} = \begin{pmatrix} 0&\gamma\\ 0&0 \end{pmatrix} \end{equation}
|
For the first evolution equation of 
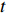
|
\begin{equation*} \begin{split} \begin{pmatrix} 0&\dfrac{\partial \gamma(t)}{\partial t}\\ 0&0 \end{pmatrix}=& \dfrac{\partial }{\partial t}(f_H^{-1}\bar{\partial }f_H) = \\& -f_{H}^{-1}\dfrac{\partial f_H}{\partial t}f_{H}^{-1}\bar{\partial}f_H+ f_{H}^{-1}\circ \bar{\partial}_E \circ f_{H}\circ f_{H}^{-1}\dfrac{\partial f_H}{\partial t}+\\ & f_{H}^{-1}\dfrac{\partial f_H}{\partial t}\circ \bar{\partial}_{S\oplus Q} =\begin{pmatrix} 0&\bar{\partial}_{S\otimes Q^{*}}\varPsi(t)\\ 0&0 \end{pmatrix}. \end{split} \end{equation*}
|
Thus, we obtain the first evolution Eq. (23).
Concerning the second evolution equation of 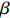
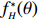
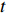
|
\begin{equation*} \begin{split} \begin{pmatrix} 0&\dfrac{\partial \beta(t)}{\partial t}\\ 0&0 \end{pmatrix} =& \frac{\partial }{\partial t}(f_{H}^*(\theta)) = \dfrac{\partial }{\partial t}(f_{H}^{-1}\circ \theta \circ f_{H})= \\ & -f_{H}^{-1}\dfrac{\partial f_H}{\partial t}f_{H}^{-1}\circ \theta \circ f_{H}+f_H^{-1}\circ \theta \circ f_{H}\circ f_{H}^{-1}\dfrac{\partial f_{H}}{\partial t} = \\ & \begin{pmatrix} 0&\theta_S\circ \varPsi(t)-\varPsi(t)\circ \theta_Q\\ 0&0 \end{pmatrix}. \end{split} \end{equation*}
|
As a result, we obtain that 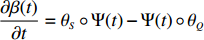
Lemma 2.2. Let 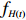
|
\tag{27} \begin{equation} f_{H_0}^{-1}f_{H(t)} = \begin{pmatrix} {\rm Id}_S&G(t)\\ 0&{\rm Id}_Q \end{pmatrix} \end{equation}
|
where 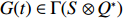
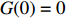
|
\tag{28} \begin{equation} \frac{\partial G}{\partial t} = 2\sqrt{-1}\varLambda_{\omega}(\partial_{S\otimes Q^{*}}\gamma+\theta_S^{*H_S}\wedge \beta+\beta\wedge \theta_Q^{*H_Q}) \end{equation}
|
and
|
\tag{29} \begin{equation} \bar{\partial}_{S\otimes Q^*}G = \gamma-\gamma_0 \end{equation}
|
Proof. Similar to the proof in Ref. [21], we have Eq. (27). For the sake of simplicity, we denote 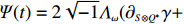
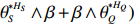
Taking the derivative of Eq. (27) with respect to t and using Eq. (22), we have that
|
\begin{equation*} \begin{split} \frac{\partial}{\partial t}(f_{H_0}^{-1}f_{H})& = f_{H_0}^{-1}f_{H}\circ f_{H}^{-1}\frac{\partial f_{H}}{\partial t} = \begin{pmatrix} 0&\varPsi(t)\\ 0&0 \end{pmatrix}. \end{split} \end{equation*}
|
As a result, we obtain Eq. (28).
Finally, taking the derivative of Eq. (28) with respect to 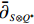
|
\tag{30} \begin{equation} \bar{\partial}_{S\otimes Q^*}\frac{\partial }{\partial t}G = \bar{\partial}_{S\otimes Q^*}\varPsi(t) = \frac{\partial}{\partial t}\gamma \end{equation}
|
Integrating Eq. (30) from 
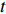
In the proof of the 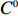
Let us recall Donaldson's functional in the Higgs bundle case:
|
\tag{31} \begin{equation} {\cal{M}}_E^0(H_0,H) = \int_0^1\int_M{\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(F_{H(s)}+[\theta,\theta^{*}])H^{-1}(s)\frac{\partial H(s)}{\partial s}\right)\frac{\omega^n}{n!}{\rm d}s \end{equation}
|
and
|
\tag{32} \begin{equation} \begin{split} {\cal{M}}_E(H_0,H)& = {\cal{M}}_E^{0}(H_0,H)-\lambda_E\int_M\log\det(H_0^{-1}H)\frac{\omega^n}{n!} = \\ & \int_0^1\int_M{\rm tr}\left((\sqrt{-1}\varLambda_{\omega}(F_{H}+[\theta,\theta^{*}])-\lambda_E{\rm Id_E})H^{-1}\frac{\partial H}{\partial s}\right)\frac{\omega^n}{n!}{\rm d}s \end{split} \end{equation}
|
where 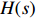
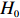
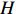
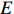

If we have an exact sequence of Higgs bundles, then
|
\tag{33} \begin{equation} 0\rightarrow (S,\theta_S)\rightarrow (E,\theta)\rightarrow (Q,\theta_Q)\rightarrow 0 \end{equation}
|
where 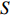
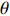

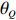

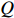
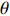
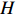
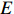

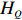
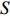
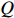

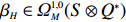
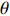
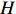
Lemma 2.3. For any exact sequence of Higgs bundles (33), Donaldson's functional 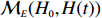
|
\tag{34} \begin{equation} \begin{split} {\cal{M}}_{E}^0(H_0,H(t))& = {\cal{M}}_{S}^0(H_{0,S},H_S(t))+ {\cal{M}}_{Q}^{0}(H_{0,Q},H_Q(t))+\\ &\quad ||\gamma(t)||_{L^2}^2-||\gamma(0)||_{L^2}^2+||\beta(t)||_{L^2}^2-||\beta(0)||_{L^2}^2 \end{split} \end{equation}
|
where 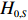
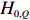

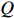
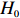

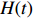
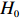
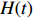
Proof. Taking derivative of 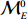
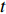
|
\tag{35} \begin{equation} \frac{{\rm d}}{{\rm d}t}{\cal{M}}_{E}^0(H_0,H(t)) = \int_M{\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(F_{H(t)}+[\theta,\theta^{*H(t)}])H^{-1}(t)\frac{\partial H(t)}{\partial t}\right)\frac{\omega^n}{n!} \end{equation}
|
Then, we obtain Donaldson's functional of the pullback metric 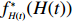
|
\begin{equation*} \begin{split} & \frac{{\rm d}}{{\rm d}t}{\cal{M}}_{E}^0(f_{H}^{*}(H_0),f_{H(t)}^{*}(H(t))) = \\ &\quad \int_M{\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(f_{H(t)}^*(F_{H(t)}+[\theta,\theta^{*H(t)}]))f_{H(t)}^*\left(H^{-1}(t)\frac{\partial H(t)}{\partial t}\right)\right)\frac{\omega^n}{n!} = \\ & \quad \int_M{\rm tr}\left\{\begin{pmatrix} \sqrt{-1}\varLambda_{\omega}(F_{H_S}+[\theta_S,\theta_S^{*}])& \sqrt{-1}\varLambda_{\omega}(\partial_{S\otimes Q^*}\gamma)\\ +\sqrt{-1}\varLambda_{\omega}(-\gamma \wedge\gamma^{*}+\beta\wedge\beta^{*})& +\sqrt{-1}\varLambda_{\omega}(\theta_S^{*}\wedge\beta+\beta\wedge \theta_Q^{*})\\ \sqrt{-1}\varLambda_{\omega}(-\bar{\partial}_{S^*\otimes Q}\gamma^{*})&\sqrt{-1}\varLambda_{\omega}(F_{H_Q}+[\theta_Q,\theta_Q^{*}])\\ +\sqrt{-1}\varLambda_{\omega}(\beta^{*H}\wedge \theta_S+\theta_Q\wedge \beta^{*})&+\sqrt{-1}\varLambda_{\omega}(-\gamma^{*}\wedge \gamma+\beta^{*}\wedge \beta) \end{pmatrix}\right. \left.\begin{pmatrix} H_{S}^{-1}\dfrac{\partial H_S}{\partial t}& -\varPsi(t)\\ -\varPsi(t)^*&H_Q^{-1}\dfrac{\partial H_Q}{\partial t} \end{pmatrix}\right\}\dfrac{\omega^n}{n!} = \end{split} \end{equation*}
|
|
\begin{equation*} \begin{split} & \quad \frac{\rm d}{{\rm d}t}{\cal{M}}_{S}^0(H_{0,S},H_S)+\frac{\rm d}{{\rm d}t}{\cal{M}}_{Q}^0(H_{0,Q},H_Q) +\\ &\quad\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left((-\gamma\wedge\gamma^*)H_{S}^{-1}\frac{\partial H_S}{\partial t}+(-\gamma^{*}\wedge \gamma)H_{Q}^{-1}\frac{\partial H_Q}{\partial t}\right)\frac{\omega^n}{n!}+\\ &\quad\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left((\partial_{S\otimes Q^*}\gamma)(-\varPsi(t)^{*})+(-\bar{\partial}_{S^*\otimes Q}\gamma^*)(-\varPsi((t))\right)\frac{\omega^n}{n!} +\\ &\quad \int_{M}\sqrt{-1}\varLambda_{\omega}{\rm tr}\left((\beta\wedge \beta^*)H_S^{-1}\frac{\partial H_S}{\partial t}+(\beta^*\wedge \beta)H_Q^{-1}\frac{\partial H_Q}{\partial t}\right)\frac{\omega^n}{n!}\ +\\ &\quad \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left((\theta_S^*\wedge \beta+\beta\wedge \theta_Q^*)(-\varPsi(t)^*)+(\beta^*\wedge \theta_S+\theta_Q\wedge \beta^*)(-\varPsi(t))\right)\frac{\omega^n}{n!} =: \\ &\quad \frac{\rm d}{{\rm d}t}{\cal{M}}_{S}^0(H_{0,S},H_S(t))+\frac{\rm d}{{\rm d}t}{\cal{M}}_{Q}^0(H_{0,Q},H_Q(t)) + I_1+I_2+I_3+I_4. \end{split} \end{equation*}
|
Recall that
|
\tag{36} \begin{equation} \left(\frac{\partial \gamma}{\partial t}\right)^{*H} = \left(\bar{\partial}_{S\otimes Q^*}\varPsi(t)\right)^{*H} = \partial_{S^*\otimes Q}\varPsi(t)^{*H} \end{equation}
|
Using Eq. (36) and Stokes formula, we have
|
\begin{equation*} \begin{split} I_2& = -\int_M\sqrt{-1}\varLambda_{\omega}\partial{\rm tr}\left(\gamma\varPsi(t)^*\right)\frac{\omega^n}{n!}-\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\gamma \wedge \partial_{S^*\otimes Q}\Psi(t)^*\right)\frac{\omega^n}{n!} +\\ &\quad \int_M\sqrt{-1}\varLambda_{\omega}\bar{\partial}{\rm tr}\left(\gamma^*\varPsi(t)\right)\frac{\omega^n}{n!}+\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\gamma^*\wedge \bar{\partial}_{S\otimes Q^*}\varPsi(t)\right)\frac{\omega^n}{n!} =\\ & -\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\gamma\wedge \left(\frac{\partial \gamma}{\partial t}\right)^*\right)\frac{\omega^n}{n!}+\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\gamma^*\wedge\left(\frac{\partial \gamma}{\partial t}\right)\right)\frac{\omega^n}{n!}. \end{split} \end{equation*}
|
Given that
|
\tag{37} \begin{equation} \begin{split} \frac{\rm d}{{\rm d}t}\gamma(t)^{*H(t)}& = \frac{\rm d}{{\rm d}t}\left(H_Q^{-1}\bar{\gamma}^{T}H_S\right) =\\ & -H_Q^{-1}\frac{\partial H_Q}{\partial t}H_Q^{-1}\bar{\gamma}^T H_S+H_Q^{-1}\overline{\left(\frac{\partial \gamma}{\partial t}\right)}^TH_S+H_Q^{-1}\bar{\gamma}^T\frac{\partial H_S}{\partial t} = \\ & \left(\frac{\partial \gamma}{\partial t}\right)^*-H_Q^{-1}\frac{\partial H_Q}{\partial t}\gamma^*+\gamma^*H_S^{-1}\frac{\partial H_S}{\partial t} \end{split} \end{equation}
|
we obtain
|
\tag{38} \begin{equation} \begin{split} &I_1+I_2 = \\ & \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(-\gamma\wedge \gamma^*H_S^{-1}\frac{\partial H_S}{\partial t}-\gamma^*\wedge \gamma H_Q^{-1}\frac{\partial H_Q}{\partial t}\right)\frac{\omega^n}{n!} +\\ &\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(-\gamma\wedge \left(\frac{\partial \gamma}{\partial t}\right)^*+\gamma^*\wedge \left(\frac{\partial \gamma}{\partial t}\right)\right)\frac{\omega^n}{n!} =\\ & \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\gamma^*H_S^{-1}\frac{\partial H_S}{\partial t}\wedge \gamma-H_Q^{-1}\frac{\partial H_Q}{\partial t}\gamma^*\wedge \gamma+\left(\frac{\partial \gamma}{\partial t}\right)^*\wedge \gamma\right)\frac{\omega^n}{n!} +\\ & \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\gamma^*\wedge \left(\frac{\partial \gamma}{\partial t}\right)\right)\frac{\omega^n}{n!} = \\ & \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\frac{\partial }{\partial t}\left(\gamma^*\right)\wedge \gamma +\gamma^*\wedge \left(\frac{\partial \gamma}{\partial t}\right)\right)\frac{\omega^n}{n!} = \\ & \frac{\rm d}{{\rm d}t}\int_M\sqrt{-1}\varLambda_{\omega}\left(\gamma^*(t)\wedge \gamma(t)\right)\frac{\omega^n}{n!} =\\ & \frac{\rm d}{{\rm d}t}||\gamma(t)||_{L^2}^2. \end{split} \end{equation}
|
Given that
|
\tag{39} \begin{equation} \frac{\partial \beta}{\partial t} = \theta_S\circ \varPsi(t)-\varPsi(t)\circ \theta_Q \end{equation}
|
We can obtain
|
\tag{40} \begin{equation} \left(\frac{\partial \beta}{\partial t}\right)^* = \varPsi^*(t)\circ \theta_S^*-\theta_Q^*\circ \varPsi^*(t) \end{equation}
|
Thus, we have
|
\begin{equation*} \begin{split} I_4 =& \int_M\sqrt{-1}\varLambda_{\omega}\left((\theta_S^*\wedge \beta+\beta\wedge \theta_Q^*)(-\varPsi^*(t))+(\beta^*\wedge \theta_S+\theta_Q\wedge \beta^*)(-\varPsi(t))\right)\frac{\omega^n}{n!} =\\ & \int_M\sqrt{-1}\varLambda_{\omega}\left(\beta\wedge(\theta_S^*\circ \varPsi^*(t)-\theta_Q^*\circ \varPsi^*(t))+(\theta_S\circ \varPsi(t)-\varPsi(t)\circ \theta_Q)\wedge \beta^*\right)\frac{\omega^n}{n!} =\\ & \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\beta\wedge \left(\frac{\partial \beta}{\partial t}\right)^*+\frac{\partial \beta}{\partial t}\wedge \beta^*\right)\frac{\omega^n}{n!}. \end{split} \end{equation*}
|
Given that
|
\tag{41} \begin{equation} \frac{\rm d}{{\rm d}t}\beta(t)^{*H(t)} = \left(\frac{\partial \beta}{\partial t}\right)^*-H_Q^{-1}\frac{\partial H_Q}{\partial t}\beta^*+\beta^*H_S^{-1}\frac{\partial H_S}{\partial t} \end{equation}
|
we obtain
|
\begin{split}
I_3+I_4& = \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left((\beta\wedge \beta^*)H_S^{-1}\frac{\partial H_S}{\partial t}+(\beta^*\wedge \beta)H_Q^{-1}\frac{\partial H_Q}{\partial t}\right)\frac{\omega^n}{n!} +\\ &\quad \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\beta\wedge \left(\frac{\partial \beta}{\partial t}\right)^*+\frac{\partial \beta}{\partial t}\wedge \beta^*\right)\frac{\omega^n}{n!} =
\end{split}
|
|
\tag{42} \begin{equation} \begin{split} \qquad \quad & \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\beta\wedge\left(\left(\frac{\partial \beta}{\partial t}\right)^*-H_Q^{-1}\frac{\partial H_Q}{\partial t}\beta^*+\beta^*H_S^{-1}\frac{\partial H_S}{\partial t}\right)\right)\frac{\omega^n}{n!} +\\ & \quad\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\left(\frac{\partial \beta}{\partial t}\right)\wedge \beta^*\right)\frac{\omega^n}{n!} =\\ & \quad \int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\beta\wedge \frac{\rm d}{{\rm d}t}\left(\beta\right)^*+\left(\frac{\partial \beta}{\partial t}\right)\wedge \beta^*\right)\frac{\omega^n}{n!} =\\ & \quad \frac{\rm d}{{\rm d}t}\int_M\sqrt{-1}\varLambda_{\omega}{\rm tr}\left(\beta(t)\wedge \beta^*(t)\right)\frac{\omega^n}{n!} =\\ & \quad \frac{\rm d}{{\rm d}t}||\beta(t)||_{L^2}^2 \end{split} \end{equation}
|
Combining Eqs. (38) and (42), we have
|
\tag{43} \begin{equation} \begin{split} &\frac{\rm d}{{\rm d}t}{\cal{M}}_{E}^0(H_0,H(t))= \\& \quad \frac{\rm d}{{\rm d}t}{\cal{M}}_{S}^0(H_{0,S},H_S(t))+ \frac{\rm d}{{\rm d}t}{\cal{M}}_{Q}^0(H_{0,Q},H_Q(t)) +\\& \quad \frac{\rm d}{{\rm d}t}||\gamma(t)||_{L^2}^2+\frac{\rm d}{{\rm d}t}||\beta(t)||_{L^2}^2 \end{split} \end{equation}
|
Integrating Eq. (43) from 
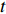
At the end of this section, we consider parabolic inequalities for 
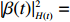
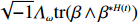
|
\tag{44} \begin{equation} \begin{split} &\left(\Delta-\frac{\partial}{\partial t}\right)|\gamma(t)|_{H(t)}^2 = \\ & \quad2|\nabla^{H(t)}\gamma|_{H(t)}^2+2 {\rm Ric}_{\omega}(\partial_k,\bar{\partial}_j)g^{k\bar{l}}g^{i\bar{j}}{\rm tr}\left(\gamma_{\bar{l}}H_{Q}^{-1}\overline{\gamma_{\bar{i}}^T}H_S\right) +\\ &\quad 4 {\rm Re}\langle g^{k\bar{l}}\left(F_{H_S}(\partial_k,\bar{\partial}_j)\gamma_{\bar{l}}-\gamma_{\bar{l}}F_{H_Q}(\partial_k,\bar{\partial}_j) \right){\rm d}\bar{z}^j,\gamma\rangle_{H(t)}-\\ &\quad 4 {\rm Re}\langle\bar{\partial}_{S\otimes Q^*}(\theta_S^*\wedge\beta+\beta\wedge \theta_Q^*),\gamma\rangle_{H(t)} +\\ &\quad 2\langle\left(-\sqrt{-1}\varLambda_{\omega}(\gamma\wedge \gamma^*-\beta\wedge \beta^*)\right)\circ \gamma + \\ &\quad \gamma\circ \left(\sqrt{-1}\varLambda_{\omega}(\gamma^*\wedge \gamma-\beta^*\wedge \beta)\right)\rangle_{H(t)} + \\ &\quad 2{\rm Re}\langle(-\sqrt{-1}\varLambda_{\omega}[\theta_S,\theta_S^*])\circ \gamma + \\ &\quad \gamma\circ(\sqrt{-1}\varLambda_{\omega}[\theta_Q,\theta_Q^*]),\gamma\rangle_{H(t)} \end{split} \end{equation}
|
and
|
\tag{45} \begin{equation} \begin{split} & \left(\Delta-\frac{\partial}{\partial t}\right)|\beta(t)|_{H(t)}^2 =\\ &\quad 2|\nabla^{H(t)}\beta(t)|_{H(t)}^2+2 {\rm Ric}_{\omega}(\partial_i,\bar{\partial_l})g^{k\bar{l}}g^{i\bar{j}}{\rm tr}\left(\beta_kH_Q^{-1}\bar{\beta_j}^TH_S\right) +\\ &\quad4{\rm Re}\langle g^{k\bar{l}}(D_{\partial_k}^{H(t)}D_{\bar{\partial}_l}^{H(t)}\beta_i){\rm d}z^i+(D_{\bar{\partial}_l}^{H(t)}\beta_i) (\nabla_{\partial_k}{\rm d}z^i,\beta)\rangle_{H(t)} -\\ &\quad4{\rm Re}\langle\theta_S\circ \varPsi(t)-\varPsi(t)\circ \theta_Q,\beta\rangle_{H(t)} +\\ &\quad2\langle\left(-\sqrt{-1}\varLambda_{\omega}(\gamma\wedge \gamma-\beta\wedge \beta^*)\right)\circ \beta+\\ &\quad \beta\circ \left(\sqrt{-1}\varLambda_{\omega}(\gamma^*\wedge \gamma-\beta^*\wedge \beta)\right),\beta\rangle_{H(t)} +\\ &\quad 2{\rm Re}\langle\sqrt{-1}\varLambda_{\omega}([\theta_S,\theta_S^*])\circ \beta-\beta \circ \sqrt{-1}\varLambda_{\omega}([\theta_Q,\theta_Q^*]),\beta\rangle_{H(t)} \end{split} \end{equation}
|
Note that the Higgs bundles 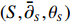
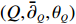
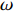
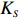
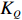

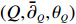
|
\tag{46} \begin{equation} \sqrt{-1}\varLambda_{\omega}(F_{K_S}+[\theta_S,\theta_S^{*K_S}]) = \lambda_S {\rm Id}_S \end{equation}
|
and
|
\tag{47} \begin{equation} \sqrt{-1}\varLambda_{\omega}(F_{K_Q}+[\theta_Q,\theta_Q^{*K_Q}]) = \lambda_Q{\rm Id}_Q \end{equation}
|
where 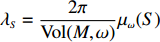

Let us denote 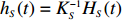
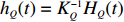

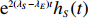
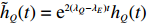
|
\tag{48} \begin{equation} \begin{split} (\Delta-\frac{\partial}{\partial t}){\rm tr}\tilde{h}_S& = 2{\rm tr}\left(-\sqrt{-1}\varLambda_{\omega}\bar{\partial}\tilde{h}_{S}\tilde{h}^{-1}_{S} \partial_{K_{S}}\tilde{h}_{S}\right)-2(\lambda_{S}-\lambda_{E}){\rm tr}(\tilde{h}_{S}) -\\ &\quad2{\rm tr}\left(\tilde{h}_{S}\sqrt{-1}\varLambda_{\omega}(F_{H_S}-F_{K_S})\right)-{\rm tr}\left(\tilde{h}_{S}H_{S}^{-1}\frac{\partial H_{S}}{\partial t}\right) =\\ & \quad2{\rm tr}\left(-\sqrt{-1}\varLambda_{\omega}\bar{\partial}\tilde{h}_{S}\tilde{h}^{-1}_{S} \partial_{K_{S}}\tilde{h}_{S}\right) -\\ &\quad2{\rm tr}(\tilde{h}_{S}(\sqrt{-1}\varLambda_{\omega}\gamma\wedge \gamma^*))+2{\rm tr}(\tilde{h}_{S}(\sqrt{-1}\varLambda_{\omega}\beta\wedge \beta^*)) +\\ &\quad 2{\rm tr}\left(\tilde{h}_{S}(\sqrt{-1}\varLambda_{\omega}[\theta_S,\theta_S^{*H_{S}} -\theta_S^{*K_{S}}])\right)\geqslant 0 \end{split} \end{equation}
|
and
|
\tag{49} \begin{equation} \begin{split} & (\Delta-\frac{\partial }{\partial t}){\rm tr}\tilde{h}^{-1}_{Q}=\\ &\quad 2{\rm tr}\left(-\sqrt{-1}\varLambda_{\omega}\bar{\partial}h_{Q}^{-1}\circ \tilde{h}_{Q}\circ \partial_{H_{Q}}\tilde{h}_{Q}^{-1}\right) + \\ &\quad 2{\rm tr} \left(\tilde{h}_{Q}^{-1}(\sqrt{-1}\varLambda_{\omega}\gamma^*\wedge \gamma)\right)-2{\rm tr}\left(\tilde{h}^{-1}_{Q}(\sqrt{-1}\varLambda_{\omega}\beta^*\wedge \beta)\right) +\\ &\quad2{\rm tr}\left(\tilde{h}^{-1}_{Q}(\sqrt{-1}\varLambda_{\omega} [\theta_Q,\theta_Q^{*K_{Q}}-\theta_Q^{*H_{Q}}])\right) \geqslant 0 \end{split} \end{equation}
|
where we have applied the non-negativity of 
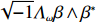
|
\tag{50} \begin{equation} {\rm tr}\left\{\tilde{h}_{S}(\sqrt{-1}\varLambda_{\omega}[\theta_S,\theta_S^{*H_{S}}- \theta_{S}^{*K_{S}}])\right\} = |\theta_S\tilde{h}_{S}^{\frac{1}{2}}-\tilde{h}_{S}\theta_S \tilde{h}_{S}^{-\frac{1}{2}}|_{K_{S}}^2 \end{equation}
|
and
|
\tag{51} \begin{equation} {\rm tr}\left\{\tilde{h}^{-1}_{Q}(\sqrt{-1}\varLambda_{\omega}[\theta_Q,\theta_Q^{*K_{Q}}- \theta_{Q}^{*H_{Q}}])\right\} = |\tilde{h}^{-\frac{1}{2}}_{Q}\theta_Q-\tilde{h}_{Q}^{\frac{1}{2}} \theta_Q\tilde{h}^{-1}_{Q}|^2_{K_{Q}} \end{equation}
|
Using inequalities (48) and (49), and the maximum principle, we can obtain a uniform bound on 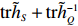
Lemma 3.1. There exists a uniform constant 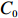
|
\tag{52} \begin{equation} \sup\limits_{x\in M}\left({\rm tr}\tilde{h}_{S}(x,t)+{\rm tr}\tilde{h}_{Q}^{-1}(x,t)\right)\leqslant C_0 \end{equation}
|
for all 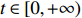
In the following, we will derive uniform upper bounds on 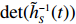
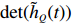
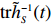
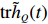
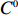

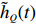
Proposition 3.2. Along the heat flow (9), we have
|
\tag{53} \begin{equation} {\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(F_{H_{S}}+[\theta_S,\theta_S^*]-\gamma \wedge \gamma^*+\beta\wedge \beta^*)-\lambda_{S} \cdot {\rm Id}_{S}\right)\leqslant v(t) \end{equation}
|
where 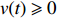
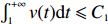

Proof. From Lemma 2.3, for any exact sequence of Higgs bundles,
|
\tag{54} \begin{equation} 0\rightarrow (S,\theta_S)\rightarrow (E,\theta)\rightarrow (Q,\theta_Q)\rightarrow 0 \end{equation}
|
we have proved that
|
\tag{55} \begin{equation} \begin{split} {\mathcal{M}}_{E}^0(H_0,H(t))& = {\cal{M}}_{S}^0(H_{S,0},H_S(t))+ {\cal{M}}_{Q}^{0}(H_{Q,0},H_Q(t))+\\& \quad ||\gamma(t)||_{L^2}^2-||\gamma(0)||_{L^2}^2+||\beta(t)||_{L^2}^2-||\beta(0)||_{L^2}^2 \end{split} \end{equation}
|
According to the flow equations (20) and (21), we have that
|
\tag{56}\begin{split} & \int_{{M}}\log\det(H_{S}^{-1}(0)H_{S}(t))\frac{\omega^n}{n!} =\\ &\quad \int_0^t\frac{\partial }{\partial l}\int_{M}\log\det(H_{S}^{-1}(0)H_{S}(l))\frac{\omega^n}{n!}{\rm d}l =\\ &\quad \int_0^t\int_{M}{\rm tr}\left(H_{S}^{-1}(l)\frac{\partial H_{S}(l)}{\partial t}\right)\frac{\omega^n}{n!}{\rm d}l = \\ &\quad-2\int_0^t\int_{M}{\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(F_{H_{S}}+[\theta_S,\theta_S^*]-\right.\\ &\quad \left.\gamma\wedge \gamma^*+\beta\wedge \beta^*)-\lambda_E {\rm Id}_S\right)\frac{\omega^n}{n!}{\rm d}l =\\ &\quad -2\int_0^t\int_{M}(|\gamma(l)|_{H(l)}^2+|\beta(l)|_{H(l)}^2)\frac{\omega^n}{n!}{\rm d}l-\\ &\quad 2(\lambda_{S}-\lambda_{E}){\rm rank}(S)t{\rm Vol}(M,\omega) \end{split}
|
and
|
\tag{57} \begin{equation} \begin{split} & \int_{M}\log\det(H_{Q}^{-1}(0)H_{Q}(t))\frac{\omega^n}{n!} =\\ &\quad 2\int_0^t\int_{M}\left(|\gamma(l)|_{H(l)}^2+|\beta(l)|_{H(l)}^2\right) \frac{\omega^n}{n!}{\rm d}l-\\ &\quad 2(\lambda_{Q}-\lambda_{E}){\rm rank}(Q)t{\rm Vol}(M,\omega) \end{split} \end{equation}
|
Then,
|
\tag{58} \begin{equation} \begin{split} {\cal{M}}_{E}(H_0,H(t))& = {\cal{M}}_{S}(H_{S,0},H_{S}(t))+{\cal{M}}_{Q}(H_{Q,0},H_{Q}(t)) +\\ &\quad||\gamma(t)||_{L^2}^2-||\gamma(0)||_{L^2}^2+||\beta(t)||_{L^2}^2-||\beta(0)||_{L^2}^2 -\\ &\quad2(\lambda_{S}-\lambda_{E})^2{\rm rank}(S)t{\rm Vol}(M,\omega) -\\ &\quad 2(\lambda_{Q}-\lambda_{E})^2{\rm rank}(Q)t{\rm Vol}(M,\omega) -\\ &\quad 2(\lambda_{S}-\lambda_{Q})\int_0^t\int_{M}\left(|\gamma(l)|_{H(l)}^2+|\beta(l)|_{H(l)}^2\right) \frac{\omega^n}{n!}{\rm d}l \end{split} \end{equation}
|
Furthermore, from the definition of Donaldson's functional and Gauss-Codazzi Eq. (17), it follows that
|
\tag{59} \begin{equation} \begin{split} {\cal{M}}_{S}&(H_{S,0},H_{S}(t))+{\cal{M}}_{Q}(H_{Q,0},H_{Q}(t)) =\\ &\quad - ||\gamma(t)||_{L^2}^2+||\gamma(0)||_{L^2}^2-||\beta(t)||_{L^2}^2+||\beta(0)||_{L^2}^2-\\ &\quad2(\lambda_{S}-\lambda_{Q})\int_0^t\int_{M}\left(|\gamma(l)|_{H(l)}^2+ |\beta(l)|_{H(l)}^2\right)\frac{\omega^n}{n!}{\rm d}l-\\ &\quad 4\int_0^t||\partial_{S\otimes Q^*}\Psi(l)||_{L^2}^2 {\rm d}l-\\ &\quad 2\int_0^t||\sqrt{-1}\Lambda_{\omega}(F_{H_{S}}+ [\theta_S,\theta_S^*]-\gamma\wedge \gamma^*+\beta\wedge \beta^*)-\\ &\quad \lambda_{S}\cdot {\rm Id}_S||_{L^2}^2 (l) {\rm d}l -\\ &\quad 2\int_0^t||\sqrt{-1}\Lambda_{\omega}(F_{H_{Q}}+ [\theta_Q,\theta_Q^*]-\gamma^*\wedge \gamma+\beta^*\wedge \beta)-\\ &\quad\lambda_{Q}\cdot {\rm Id}_{Q}||_{L^2}^2(l){\rm d}l \end{split} \end{equation}
|
Now, we set
|
\tag{60} \begin{equation} \begin{split} \tilde{v}(t)& = 2||\partial_{S\otimes Q^*}\varPsi(t)||_{L^2}^2+(\lambda_{S}-\lambda_{Q})\left(||\gamma(t)||_{L^2}^2+ ||\beta(t)||_{L^2}^2\right) +\\ &\quad||\sqrt{-1}\varLambda_{\omega}(F_{H_{S}}+[\theta_S,\theta_S^*]-\gamma\wedge \gamma^*+\beta \wedge \beta^*)-\lambda_{S}\cdot{\rm Id}_S||_{L^2}^2(t) +\\ &\quad||\sqrt{-1}\varLambda_{\omega}(F_{H_{Q}}+[\theta_Q,\theta_Q^*]-\gamma^*\wedge \gamma+\beta^*\wedge \beta)-\lambda_{Q}\cdot{\rm Id}_Q||_{L^2}^2(t) \end{split} \end{equation}
|
Given that 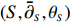

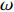
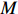
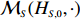
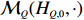
|
\tag{61} \begin{equation} \int_0^{+\infty}\tilde{v}(t){\rm d}t\leqslant \tilde{C}_1<+\infty \end{equation}
|
where 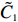
By contrast, along with the heat flow Eq. (9), we have
|
\tag{62} \begin{equation} \left(\Delta-\frac{\partial}{\partial t}\right)|\sqrt{-1}\varLambda_{\omega}(F_{H(t)}+[\theta,\theta^*])-\lambda_{E} {\rm Id}_{E}|_{H(t)}^2 \geqslant 0 \end{equation}
|
According to the estimate of the heat kernel 
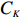
|
\tag{63} \begin{equation} 0<K(x,y,t)\leqslant C_{K}t^{-n} \end{equation}
|
Applying the maximum principle, we have
|
\tag{64} \begin{equation} \begin{split} &\quad |\sqrt{-1}\varLambda_{\omega}(F_{H}(t)+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}|_{H(t)}^2(x,t+s) \leqslant \\ & \int_{M}K(x,y,s)|\sqrt{-1}\varLambda_{\omega}(F_{H(t)}+[\theta,\theta^*])- \lambda_{E}{\rm Id}_{E}|_{H}^2(y,t)\frac{\omega^n}{n!}(y) \leqslant \\ & C_{K}s^{-n}\int_{M}|\sqrt{-1}\varLambda_{\omega} (F_{H(t)}+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}|_{H(t)}^2(y,t)\frac{\omega^n}{n!}(y) \end{split} \end{equation}
|
for any 
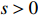
|
\tag{65} \begin{equation} \begin{split} & 2(\lambda_{S}-\lambda_{Q})\;\cdot \\ &\quad{\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(F_{H_{S}}+[\theta_S,\theta_S^*]-\gamma\wedge \gamma^*+\beta\wedge \beta^*)-\lambda_{S}{\rm Id}_S\right)(t)\leqslant \\ &\quad |\sqrt{-1}\varLambda_{\omega}(F_{H(t)}+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}|_{H(t)}^2 -\\ &\quad (\lambda_{S}-\lambda_{E})^2{\rm rank}(S)-(\lambda_{Q}-\lambda_{E})^2{\rm rank}(Q) -\\ &\quad2(\lambda_{Q}-\lambda_{E}){\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(F_{H(t)}+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}\right) \leqslant \\ &\quad C_{K}\left(\frac{t}{2}\right)^{-n}\int_{M}|\sqrt{-1} \varLambda_{\omega}(F_{H(\frac{t}{2})}+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}|_{H(\frac{t}{2})}^2 -\\ &\quad(\lambda_{S}-\lambda_{E}){\rm rank}(S)-(\lambda_{Q}-\lambda_{E})^2{\rm rank}(Q) +\\ &\quad(\lambda_{E}-\lambda_{Q})|{\rm tr}\left(\sqrt{-1}\Lambda_{\omega}(F_{H(t)}+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}\right)| \leqslant \\ &\quad C_{K}\left(\frac{t}{2}\right)^{-n}\tilde{v}\left(\frac{t}{2}\right)+\\ &\quad 2(\lambda_{E}-\lambda_{Q})|{\rm tr}\left(\sqrt{-1}\Lambda_{\omega}(F_{H(t)}+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}\right)| +\\ &\quad C_K\left(\frac{t}{2}\right)^{-n}\{(\lambda_{S}-\lambda_{E})^2{\rm rank}(S)+(\lambda_{Q}-\lambda_{E})^2{\rm rank}(Q)\} \end{split} \end{equation}
|
Let us set
|
\tag{66} \begin{equation} \begin{split} v(t)& = (2(\lambda_{S}-\lambda_{Q}))^{-1}\left\{C_{K}\left(\frac{t}{2}\right)^{-n}\tilde{v} \left(\frac{t}{2}\right)\right. +\\ &\quad 2(\lambda_{E}-\lambda_{Q})\max\limits_{x\in M}|{\rm tr}\left(\sqrt{-1}\Lambda_{\omega}(F_{H(t)}+[\theta,\theta^*])-\lambda_{E}{\rm Id}_{E}\right)| +\\ &\quad \left.C_K\left(\frac{t}{2}\right)^{-n}\{(\lambda_{S}-\lambda_{E})^2{\rm rank}(S)+(\lambda_{Q}-\lambda_{E})^2{\rm rank}(Q)\}\right\} \end{split} \end{equation}
|
Combining formulas (61) and (65), and noting that 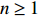
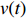
Using Proposition 3.2, we obtain a uniform 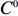

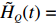
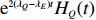
Theorem 3.3. Let 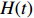

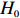
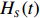
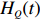
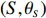
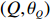
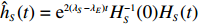
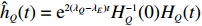
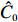
|
\tag{67} \begin{equation} \sup\limits_{x\in M}\{{\rm tr}\hat{h}_{S}(x,t)+{\rm tr}\hat{h}^{-1}_{S}(x,t)+ {\rm tr}\hat{h}_{Q}(x,t)+ {\rm tr}\hat{h}^{-1}_{Q}(x,t) \}\leqslant \hat{C}_0 \end{equation}
|
for all 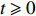
Proof. Noting that the metrics 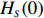
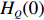
|
\tag{68} \begin{equation} -C_{1}{\rm Id}_S \leqslant \sqrt{-1}\varLambda_{\omega}(F_{H_{S}(0)}+[\theta_S,\theta_S^*])\leqslant C_{1}{\rm Id}_S \end{equation}
|
and
|
\tag{69} \begin{equation} -C_{1}{\rm Id}_Q\leqslant \sqrt{-1}\varLambda_{\omega}(F_{H_{Q}(0)}+[\theta_Q,\theta_Q^*])\leqslant C_{1}{\rm Id}_Q \end{equation}
|
for the entire 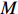
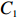
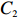
|
\tag{70} \begin{equation} \Delta\log\left({\rm tr}\tilde{h}_{S}(0)+{\rm tr}\tilde{h}_{S}^{-1}(0)\right)\geqslant -C_{2} \end{equation}
|
and
|
\tag{71} \begin{equation} \Delta\log\left({\rm tr}\tilde{h}_{Q}(0)+{\rm tr}\tilde{h}_{Q}^{-1}(0)\right)\geqslant -C_{2} \end{equation}
|
According to formulas (70), (71) and Moser's iteration, we have the following mean inequalities, including a uniform constant 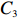
|
\tag{72} \begin{equation} \sup\limits_{M}\log\left({\rm tr}\tilde{h}_{S}(0)+{\rm tr}\tilde{h}_{S}^{-1}(0)\right) \leqslant C_{3}\int_{M}\log\left({\rm tr}\tilde{h}_{S}(0)+{\rm tr}\tilde{h}_{S}^{-1}(0)\right)\frac{\omega^n}{n!} \end{equation}
|
and
|
\tag{73} \begin{equation} \sup\limits_{M}\log\left({\rm tr}\tilde{h}_{Q}(0)+{\rm tr}\tilde{h}_{Q}^{-1}(0)\right) \leqslant C_{3}\int_{M}\log\left({\rm tr}\tilde{h}_{Q}(0)+{\rm tr}\tilde{h}_{Q}^{-1}(0)\right)\frac{\omega^n}{n!} \end{equation}
|
From the uniform 
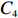
|
\tag{74} \begin{equation} \sup\limits_{M}\{\log({\rm tr}\tilde{h}_{S}(0)+{\rm tr}\tilde{h}^{-1}_{S}(0))+\log({\rm tr}\tilde{h}_{Q}(0)+{\rm tr}\tilde{h}_{Q}^{-1}(0))\}\leqslant C_{4} \end{equation}
|
Set
|
\begin{equation*} \hat{h}_{S}(t) = {\rm e}^{2(\lambda_{S}-\lambda_{E})t}H_{S}^{-1}(0)H_{S}(t) \end{equation*}
|
and
|
\begin{equation*} \hat{h}_{Q}(t) = {\rm e}^{2(\lambda_{Q}-\lambda_{E})t}H_{Q}^{-1}(0)H_{Q}(t). \end{equation*}
|
From (52) and (74), we can obtain that
|
\tag{75} \begin{equation} \sup\limits_{(x,t)\in M \times [0,+\infty)}\left({\rm tr}\hat{h}_{S}(x,t)+{\rm tr}\hat{h}_{Q}(x,t)\right)\leqslant C_{5} \end{equation}
|
where 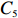
|
\tag{76} \begin{equation} \frac{\partial}{\partial t}\log\det(\hat{h}_{S}^{-1}) =\\ 2{\rm tr} \left(\sqrt{-1}\varLambda_{\omega}(F_{H_{S}}+[\theta_S,\theta_S^*]-\gamma\wedge \gamma^*+\beta\wedge \beta^*)-\lambda_{S}{\rm Id}_S\right) \end{equation}
|
From (62), the Gauss-Codazzi equation (17), and the maximum principle, we have
|
\tag{77} \begin{equation} \sup\limits_{(x,t)\in M\times[0,+\infty)}|\sqrt{-1}\varLambda_{\omega}(F_{H(t)}+[\theta,\theta^*])- \lambda_{E}{\rm Id}_{E}|_{H(t)}^2 \leqslant C_6 \end{equation}
|
and
|
\tag{78} \begin{equation} \sup\limits_{(x,t)\in M \times [0,+\infty)}|{\rm tr}\left(\sqrt{-1}\varLambda_{\omega}(F_{H_{S}}+[\theta_S,\theta_S^*]-\gamma\wedge \gamma^*+\beta\wedge \beta^*)-\lambda_{S}{\rm Id}_S\right)|\leqslant C_6 \end{equation}
|
where 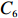

|
\tag{79} \begin{equation} \sup\limits_{(x,t)\in M\times [0,+\infty)}\log\left(\det\hat{h}_{S}^{-1}(x,t)\right)\leqslant C_{7} \end{equation}
|
As a result, we have
|
\tag{80} \begin{equation} \det(\hat{h}_{S}(t))\det(\hat{h}_{Q}(t)) = \det(H_0^{-1}H(t)) \end{equation}
|
and then
|
\tag{81} \begin{equation} \begin{split} \log\det(\hat{h}_{Q}(x,t))& = \log\det(\hat{h}_{S}^{-1}(x,t))+\log\det(H_0^{-1}H(x,t))\leqslant C_{8} \end{split} \end{equation}
|
for all 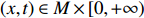

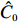
|
\tag{82} \begin{equation} \sup\limits_{(x,t)\in M \times[0,+\infty)}\left({\rm tr}\hat{h}_{S}+{\rm tr}\hat{h}_{S}^{-1}+{\rm tr}\hat{h}_{Q}+{\rm tr}\hat{h}_{Q}^{-1}\right)(x,t)\leqslant \hat{C}_0 \end{equation}
|
Using the above uniform 

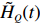
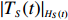

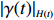
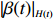
Let us set
|
\tag{83} \begin{equation} T_S(t) = D_{H_S(t),\bar{\partial}_S}-D_{H_0,\bar{\partial}_S} = h_S^{-1}\partial_{H_{0,S}}h_S = (\partial_{H_S(t)}h_S)h_S^{-1} \end{equation}
|
and
|
\tag{84} \begin{equation} T_Q(t) = D_{H_Q(t),\bar{\partial}_Q}-D_{H_0,\bar{\partial}_Q} = h_Q^{-1}\partial_{H_{0,Q}}h_Q = (\partial_{H_Q(t)}h_Q)h_Q^{-1} \end{equation}
|
where 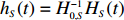
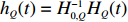
|
\tag{85} \begin{equation} \begin{split} &\bigg(\Delta-\frac{\partial}{\partial t}\bigg)|T_S|_{H_S}^2 =\\ & \quad 2|\nabla^{H_S(t)}T_S|_{H_S(t)}^2+2{\rm Ric}_{\omega}(\partial_k,\bar{\partial}_s)g^{k\bar{i}}g^{l\bar{s}}{\rm tr}\big(T_{S}(\partial_l)H_S^{-1}\overline{T_{S}(\partial_i)^T}H_S\big) +\\ &\quad 2{\rm Re}\langle[\sqrt{-1}\varLambda_{\omega}([\theta_S,\theta_S^*]-\gamma\wedge \gamma^*+\beta\wedge \beta^*),T_S],T_S\rangle_{H_S(t)} +\\ &\quad 4{\rm Re}\langle\partial_{H_S}\big(\sqrt{-1}\varLambda_{\omega}([\theta_S,\theta_S^*]-\gamma\wedge \gamma^*+\beta\wedge \beta^*)\big),T_S\rangle_{H_S(t)} +\\ &\quad 4{\rm Re}\bigg\{g^{i\bar{j}}g^{k\bar{l}}\langle[T_{S}(\partial_i),F_{H_{0,S}}(\partial_k,\bar{\partial}_j)],T_S(\partial_l)\rangle_{H_S(t)}\bigg\} +\\ &\quad 4{\rm Re}\big\langle\partial_{H_{0,S}}(\sqrt{-1}\varLambda_{\omega}F_{H_{0,S}}),T_S\big\rangle_{H_S(t)} \end{split} \end{equation}
|
and
|
\tag{86} \begin{equation} \begin{split} &\bigg(\Delta-\frac{\partial}{\partial t}\bigg)|T_Q|_{H_Q}^2 =\\ &\quad 2|\nabla^{H_Q(t)}T_Q|_{H_Q(t)}^2+2{\rm Ric}_{\omega}(\partial_k,\bar{\partial}_s)g^{k\bar{i}}g^{l\bar{s}}{\rm tr}\big(T_{Q}(\partial_l)H_Q^{-1}\overline{T_{Q}(\partial_i)^T}H_Q\big) +\\ &\quad 2{\rm Re}\langle[\sqrt{-1}\varLambda_{\omega}([\theta_Q,\theta_Q^*]-\gamma^*\wedge \gamma+\beta^*\wedge \beta),T_Q],T_Q\rangle_{H_Q(t)} +\\ &\quad 4{\rm Re}\langle\partial_{H_Q}\big(\sqrt{-1}\varLambda_{\omega}([\theta_Q,\theta_Q^*]-\gamma^*\wedge \gamma+\beta^*\wedge \beta)\big),T_Q\rangle_{H_Q(t)} +\\ &\quad 4{\rm Re}\bigg\{g^{i\bar{j}}g^{k\bar{l}}\langle[T_{Q}(\partial_i),F_{H_{0,Q}}(\partial_k,\bar{\partial}_j)],T_Q(\partial_l)\rangle_{H_Q(t)}\bigg\} +\\ &\quad4{\rm Re}\big\langle\partial_{H_{0,Q}}(\sqrt{-1}\varLambda_{\omega}F_{H_{0,Q}}),T_Q\big\rangle_{H_Q(t)} \end{split} \end{equation}
|
However, we can also obtain the following inequality (see (2.5) in Ref. [14] for further details):
|
\tag{87} \begin{equation} \bigg(\Delta-\frac{\partial}{\partial t}\bigg)|\theta|_{H(t)}^2\geqslant 2|\nabla_{H(t)}\theta|^2_{H(t)}+2|\Lambda_{\omega}[\theta,\theta^{*H(t)}]|_{H(t)}^2-2|{\rm Ric}(\omega)||\theta|_{H(t)}^2 \end{equation}
|
From the local 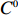

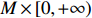
|
\tag{88} \begin{equation} \sup\limits_{M\times [0,+\infty)}|\theta|_{H(t)}^2 = \sup\limits_{M\times [0,+\infty)}\big(|\theta_S|_{H_S(t)}^2+|\theta_Q|_{H_Q(t)}^2+|\beta(t)|_{H(t)}^2\big)<\tilde{C}_1 \end{equation}
|
Together with the local uniform 
Proposition 4.1. Let 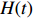


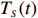
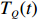
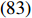
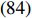
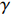
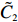
|
\tag{89} \begin{equation} \sup\limits_{(x,t)\in M\times[0,+\infty)}\big(|T_S(t)|_{H_{S}(t)}^2+|T_{Q}(t)|_{H_Q(t)}^2+|\gamma(t)|_{H(t)}^2+|\theta|_{H(t)}^2\big)(x,t) < \tilde{C}_{2} \end{equation}
|
According to Eqs. (44), (85), and (86), we obtain
|
\tag{90} \begin{equation} \begin{split} & \bigg(\Delta-\frac{\partial}{\partial t}\bigg)\big(|T_S|_{H_S(t)}^2+|T_Q|_{H_Q(t)}^2+|\gamma(t)|_{H(t)}^2\big) \geqslant \\ &\quad \frac{1}{4}\big(|F_{H_S}|_{H_S}^2+|F_{H_Q}^2|+2|\partial_{H(t)}\gamma|_{H(t)}^2\big) -\\ &\quad \tilde{C}_3\big(|\gamma|_{H(t)}^2+|\theta|_{H(t)}^2\big)\big(|T_S|_{H_S(t)}^2+|T_Q|_{H_Q(t)}^2+|\gamma(t)|_{H(t)}^2+|\theta|_{H(t)}^2\big) -\\ &\quad\tilde{C}_4\big(|T_S|_{H_S(t)}^2+|T_Q|_{H_Q(t)}^2+|\gamma(t)|_{H(t)}^2+|\theta|_{H(t)}^2\big)-\tilde{C}_5 \end{split} \end{equation}
|
where constant 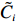
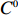
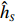

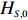

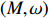
|
\tag{91} \begin{equation} \varXi(x,t) = |\nabla_{H(t)}\big(F_{H(t)}+[\theta,\theta^{*H(t)}]\big)|_{H(t)}^2+|\nabla_{H(t)}\theta|_{H(t)}^2(x) \end{equation}
|
Then, it follows that
|
\tag{92} \begin{equation} \begin{split} &\bigg(\Delta-\frac{\partial}{\partial t}\bigg)\varXi \geq \\ &\quad 2\big(|\nabla^{H(t)}(F_{H(t)}+[\theta,\theta^{*H}])|_{H(t)}^2+|\nabla_{H(t)}^2\theta|_{H(t)}^2\big)-\tilde{C}_6 \varXi^{\frac{3}{2}}-\\ &\quad \tilde{C}_6(|\theta|_{H(t)}^2+|Rm(\omega)|)\varXi(x,t)-\tilde{C}_6|\nabla_{\omega}{\rm Ric}(\omega)|^2 \end{split} \end{equation}
|
where 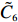
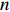
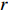
Proof of Theorem 1.1. For the sake of simplicity, we denote
|
\tag{93} \begin{equation} \nu = |\theta|_{H(t)}^2+|\gamma|_{H(t)}^2+|T_S|_{H_S(t)}^2+|T_Q|_{H_Q(t)}^2 \end{equation}
|
By estimating (89), we can choose a constant 
|
\tag{94} \begin{equation} 0\leqslant \frac{1}{2}\tilde{C}_7\leqslant \tilde{C}_7-\nu(x,t)\leqslant \tilde{C}_7 \end{equation}
|
for all 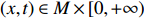
Now we consider the following test function:
|
\tag{95} \begin{equation} \zeta_1 = \frac{\varXi}{\tilde{C}_7-\nu}+W\nu \end{equation}
|
Let 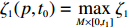
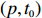
|
\tag{96} \begin{equation} \begin{split} \bigg(\Delta-\frac{\partial}{\partial t}\bigg)\zeta_1& = \frac{1}{\tilde{C}_7-\nu}\bigg(\Delta-\frac{\partial}{\partial t}\bigg)\varXi+\frac{\varXi}{(\tilde{C}_7-\nu)^2}\bigg(\Delta-\frac{\partial}{\partial t}\bigg)\nu -\\ &\quad \frac{2}{\tilde{C}_7-\nu}\nabla\bigg(\frac{\varXi}{\tilde{C}_7-\nu}\bigg)\cdot\nabla(\tilde{C}_7-\nu)+W\bigg(\Delta-\frac{\partial}{\partial t}\bigg)\nu \end{split} \end{equation}
|
and
|
\tag{97} \begin{equation} \nabla\bigg(\frac{\varXi}{\tilde{C}_7-\nu}\bigg)+W\nabla \nu = 0 \end{equation}
|
Substituting (97) into (96), choosing the constants 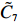

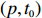
|
\tag{98} \begin{equation} \begin{split} \bigg(\Delta-\frac{\partial}{\partial t}\bigg)\zeta_1& = \frac{1}{\tilde{C}_7-\nu}\bigg(\Delta-\frac{\partial}{\partial t}\bigg)\varXi+\frac{\varXi}{(\tilde{C}_7-\nu)^2}\bigg(\Delta-\frac{\partial}{\partial t}\bigg)\nu -\\ &\quad \frac{2W}{\tilde{C}_7-\nu}|\nabla \nu|^2+W\bigg(\Delta-\frac{\partial}{\partial t}\bigg)\nu \geqslant \varXi-\tilde{C}_8 \end{split} \end{equation}
|
where 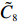

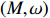
|
\tag{99} \begin{equation} \varXi(p,t_0)\leqslant \tilde{C}_8 \end{equation}
|
Then, there exists a constant 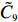
|
\tag{100} \begin{equation} \sup\limits_{M \times[0,+\infty)}|F_{H(t)}+[\theta,\theta^*]|^2+|\nabla_{H(t)}\theta|^2_{H(t)}\leqslant \tilde{C}_9 \end{equation}
|
This completes the proof of Theorem 1.1.
The author would like to express his gratitude to Prof. Xi Zhang for his advice on this study, which is supported by the National Key Research and Development Program of China (2020YFA0713100), the National Natural Science Foundation of China (12141104, 11801535, 11721101, 11625106), and the Fundamental Research Funds for the Central Universities.
The author declares that they have no conflict of interest.
The authors declare that they have no conflict of interest.
| [1] |
Hitchin N J. The self-duality equations on a Riemann surface. Proc. London Math. Soc., 1987, (55): 59–126. DOI: 10.1112/plms/s3-55.1.59
|
| [2] |
Simpson C T. Constructing variations of Hodge structure using Yang-Mills theory and applications to uniformazation. J. Amer. Math. Soc., 1988, 1: 867–918. DOI: 10.1090/S0894-0347-1988-0944577-9
|
| [3] |
Simpson C T. Higgs bundles and local systems. Inst. Hautes Études Sci. Publ. Math., 1992, 75: 5–95. DOI: 10.1007/BF02699491
|
| [4] |
Bando S, Siu Y T. Stable sheaves and Einstein-Hermitian metrics. In: Geometry and Analysis on Complex Manifolds. Singapore: World Scientific, 1994: 39-50.
|
| [5] |
Bartolomeis P D, Tian G. Stability of complex vector bundles. J. Differ. Geom., 1996, 43: 232–275. DOI: 10.4310/jdg/1214458107
|
| [6] |
Biquard O. On parabolic bundles over a complex surface. J. London Math. Soc., 1996, 53 (2): 302–316. DOI: 10.1112/jlms/53.2.302
|
| [7] |
Bradlow S B. Vortices in holomorphic line bundles over closed Kähler manifolds. Commun. Math. Phys., 1990, 135: 1–17. DOI: 10.1007/BF02097654
|
| [8] |
Bradlow S B, Garcia-Prada O. Stable triples equivariant bundles and dimensional reduction. Math. Ann., 1996, 135: 225–252. DOI: 10.1007/BF01446292
|
| [9] |
Álvarez-Cónsul L, Garcís-Prada O. Dimensional reduction,
 |
| [10] |
Shen Z, Zhang P. Canonical metrics on holomorphic filtrations over compact Hermitian manifolds. Commun. Math. Stat., 2020, 8: 219–237. DOI: 10.1007/s40304-019-00199-y
|
| [11] |
Garcia-Prada O. Dimensional reduction of stable bundles, vortices and stable pairs. Int. J. Math., 1994, 5: 1–52. DOI: 10.1142/S0129167X94000024
|
| [12] |
Huybrechts D, Lehn M. Stable pairs on curves and surfaces. J. Algebr. Geom., 1995, 4 (1): 67–104.
|
| [13] |
Atiyah M, Bott R. The Yang-Mills equations over Riemann surfaces. Philosophical Transactions of the Royal Society of London. Series A, Mathematical and Physical Sciences, 1982, 308: 524–615. DOI: 10.1098/rsta.1983.0017
|
| [14] |
Li J Y, Zhang X. The gradient flow of Higgs pairs. J. Eur. Math. Soc., 2011, 13 (5): 1373–1422. DOI: 10.4171/JEMS/284
|
| [15] |
Uhlenbeck K K. A priori estimates for Yang-Mills fields, unpublished manuscript. http://www.math.uwaterloo.ca/~karigian/ uhlenbeck-preprint.pdf.
|
| [16] |
Uhlenbeck K K. Connections with L p bounds on curvature. Commun. Math. Phys., 1982, 83 (1): 31–42. DOI: 10.1007/BF01947069
|
| [17] |
Jacob A. The Yang-Mills flow and the Atiyah-Bott formula on compact Kähler manifolds. Amer. J. Math., 2016, 138 (2): 329–365. DOI: 10.1353/ajm.2016.0011
|
| [18] |
Sibley B. Asymtotics of the Yang-Mills flow for holomorphic vector bundles over Kähler manifolds: The canonical structure of the limit. J. Reine Agnew. Math., 2015, 706: 123–191. DOI: 10.1515/crelle-2013-0063
|
| [19] |
Daskalopoulos G, Wentworth R. On the blow-up set of the Yang-Mills flow on Kähler surfaces. Math. Z., 2007, 256: 301–310. DOI: 10.1007/s00209-006-0075-2
|
| [20] |
Sibley B, Wentworth R. Analytic cycles, Bott-Chern forms, and singular sets for the Yang-Mills flow on Kähler manifolds. Adv. Math., 2015, 279: 501–531. DOI: 10.1016/j.aim.2015.04.009
|
| [21] |
Li J Y, Zhang C J, Zhang X. A note on curvature estimate of the Hermitian-Yang-Mills flow. Commun. Math. Stat., 2018, 6: 319–358. DOI: 10.1007/s40304-018-0135-z
|
| [22] |
Uhlenbeck K K, Yau S T. On existence of Hermitian-Yang-Mills connection in stable vector bundles. Comm. Pure Appl. Math., 1986, 39S: 257–293. DOI: 10.1002/cpa.3160390714
|
| [23] |
Donaldson S K. Anti-self-dual Yang-Mills connections over complex algebraic surfaces and stable vector bundles. Proc. London Math. Soc., 1985, 50: 1–26. DOI: 10.1112/plms/s3-50.1.1
|
| [24] |
Cheng S Y, Li P. Heat kernel estimates and lower bound of eigenvalues. Comment. Math. Helv., 1981, 56: 327–338. DOI: 10.1007/BF02566216
|
| [25] |
Bando S, Mabuchi T. Uniquessness of Einstein Kähler metrics modulo connected group actions. Advanced Studies in Pure Mathematics, 1987, 10: 11–40. DOI: 10.2969/ASPM/01010011
|
| [26] |
Li J Y, Zhang C J, Zhang X. Semi-stable Higgs sheaves and Bogomolov type inequality. Calc. Var. Partial Differ. Equ., 2017, 56 (3): 81. DOI: 10.1007/s00526-017-1174-0
|
| [1] |
Hitchin N J. The self-duality equations on a Riemann surface. Proc. London Math. Soc., 1987, (55): 59–126. DOI: 10.1112/plms/s3-55.1.59
|
| [2] |
Simpson C T. Constructing variations of Hodge structure using Yang-Mills theory and applications to uniformazation. J. Amer. Math. Soc., 1988, 1: 867–918. DOI: 10.1090/S0894-0347-1988-0944577-9
|
| [3] |
Simpson C T. Higgs bundles and local systems. Inst. Hautes Études Sci. Publ. Math., 1992, 75: 5–95. DOI: 10.1007/BF02699491
|
| [4] |
Bando S, Siu Y T. Stable sheaves and Einstein-Hermitian metrics. In: Geometry and Analysis on Complex Manifolds. Singapore: World Scientific, 1994: 39-50.
|
| [5] |
Bartolomeis P D, Tian G. Stability of complex vector bundles. J. Differ. Geom., 1996, 43: 232–275. DOI: 10.4310/jdg/1214458107
|
| [6] |
Biquard O. On parabolic bundles over a complex surface. J. London Math. Soc., 1996, 53 (2): 302–316. DOI: 10.1112/jlms/53.2.302
|
| [7] |
Bradlow S B. Vortices in holomorphic line bundles over closed Kähler manifolds. Commun. Math. Phys., 1990, 135: 1–17. DOI: 10.1007/BF02097654
|
| [8] |
Bradlow S B, Garcia-Prada O. Stable triples equivariant bundles and dimensional reduction. Math. Ann., 1996, 135: 225–252. DOI: 10.1007/BF01446292
|
| [9] |
Álvarez-Cónsul L, Garcís-Prada O. Dimensional reduction,
 |
| [10] |
Shen Z, Zhang P. Canonical metrics on holomorphic filtrations over compact Hermitian manifolds. Commun. Math. Stat., 2020, 8: 219–237. DOI: 10.1007/s40304-019-00199-y
|
| [11] |
Garcia-Prada O. Dimensional reduction of stable bundles, vortices and stable pairs. Int. J. Math., 1994, 5: 1–52. DOI: 10.1142/S0129167X94000024
|
| [12] |
Huybrechts D, Lehn M. Stable pairs on curves and surfaces. J. Algebr. Geom., 1995, 4 (1): 67–104.
|
| [13] |
Atiyah M, Bott R. The Yang-Mills equations over Riemann surfaces. Philosophical Transactions of the Royal Society of London. Series A, Mathematical and Physical Sciences, 1982, 308: 524–615. DOI: 10.1098/rsta.1983.0017
|
| [14] |
Li J Y, Zhang X. The gradient flow of Higgs pairs. J. Eur. Math. Soc., 2011, 13 (5): 1373–1422. DOI: 10.4171/JEMS/284
|
| [15] |
Uhlenbeck K K. A priori estimates for Yang-Mills fields, unpublished manuscript. http://www.math.uwaterloo.ca/~karigian/ uhlenbeck-preprint.pdf.
|
| [16] |
Uhlenbeck K K. Connections with L p bounds on curvature. Commun. Math. Phys., 1982, 83 (1): 31–42. DOI: 10.1007/BF01947069
|
| [17] |
Jacob A. The Yang-Mills flow and the Atiyah-Bott formula on compact Kähler manifolds. Amer. J. Math., 2016, 138 (2): 329–365. DOI: 10.1353/ajm.2016.0011
|
| [18] |
Sibley B. Asymtotics of the Yang-Mills flow for holomorphic vector bundles over Kähler manifolds: The canonical structure of the limit. J. Reine Agnew. Math., 2015, 706: 123–191. DOI: 10.1515/crelle-2013-0063
|
| [19] |
Daskalopoulos G, Wentworth R. On the blow-up set of the Yang-Mills flow on Kähler surfaces. Math. Z., 2007, 256: 301–310. DOI: 10.1007/s00209-006-0075-2
|
| [20] |
Sibley B, Wentworth R. Analytic cycles, Bott-Chern forms, and singular sets for the Yang-Mills flow on Kähler manifolds. Adv. Math., 2015, 279: 501–531. DOI: 10.1016/j.aim.2015.04.009
|
| [21] |
Li J Y, Zhang C J, Zhang X. A note on curvature estimate of the Hermitian-Yang-Mills flow. Commun. Math. Stat., 2018, 6: 319–358. DOI: 10.1007/s40304-018-0135-z
|
| [22] |
Uhlenbeck K K, Yau S T. On existence of Hermitian-Yang-Mills connection in stable vector bundles. Comm. Pure Appl. Math., 1986, 39S: 257–293. DOI: 10.1002/cpa.3160390714
|
| [23] |
Donaldson S K. Anti-self-dual Yang-Mills connections over complex algebraic surfaces and stable vector bundles. Proc. London Math. Soc., 1985, 50: 1–26. DOI: 10.1112/plms/s3-50.1.1
|
| [24] |
Cheng S Y, Li P. Heat kernel estimates and lower bound of eigenvalues. Comment. Math. Helv., 1981, 56: 327–338. DOI: 10.1007/BF02566216
|
| [25] |
Bando S, Mabuchi T. Uniquessness of Einstein Kähler metrics modulo connected group actions. Advanced Studies in Pure Mathematics, 1987, 10: 11–40. DOI: 10.2969/ASPM/01010011
|
| [26] |
Li J Y, Zhang C J, Zhang X. Semi-stable Higgs sheaves and Bogomolov type inequality. Calc. Var. Partial Differ. Equ., 2017, 56 (3): 81. DOI: 10.1007/s00526-017-1174-0
|
ISSN 0253-2778
CN 34-1054/N
Copyright © Editorial Office of JUSTC, All Rights Reserved. 皖ICP备05002528号
Supported by: Beijing Renhe Information Technology Co., Ltd. 




 DownLoad:
DownLoad: