ISSN 0253-2778

CN 34-1054/N

open
Free to Publish. Free to Read.
Open AccessOpen Access JUSTC Mathematics/Management Article 24 June 2022

Worst-case conditional value-at-risk and conditional expected shortfall based on covariance information

  • Department of Statistics and Finance, School of Management, University of Science and Technology of China, Hefei 230026, China

Cite this: JUSTC, 2022, 52(5): 4
https://doi.org/10.52396/JUSTC-2022-0023
CSTR: 32290.14.JUSTC-2022-0023
More Information
  • Author Bio:

    Tiantian Mao is an Associate Professor with the University of Science and Technology of China (USTC). In 2012, she received her Ph.D. degree in Science from USTC. In May of the same year, she joined the Department of Statistics and Finance, School of Management, USTC, for postdoctoral work. Her research fields include risk measurement, risk management, random dominance and extreme value theory

    Qinyu Wu is currently a Ph.D. candidate at the School of Management, University of Science and Technology of China. His research interests focus on risk management and mathematical finance

  • Corresponding author:

    Qinyu Wu, E-mail: wu051555@mail.ustc.edu.cn

  • Received Date: January 24, 2022
  • Accepted Date: March 29, 2022
  • Available Online: June 24, 2022
    Abstract

  • In this paper, we study the worst-case conditional value-at-risk (CoVaR) and conditional expected shortfall (CoES) in a situation where only partial information on the underlying probability distribution is available. In the case of the first two marginal moments are known, the closed-form solution and the value of the worst-case CoVaR and CoES are derived. The worst-case CoVaR and CoES under mean and covariance information are also investigated.

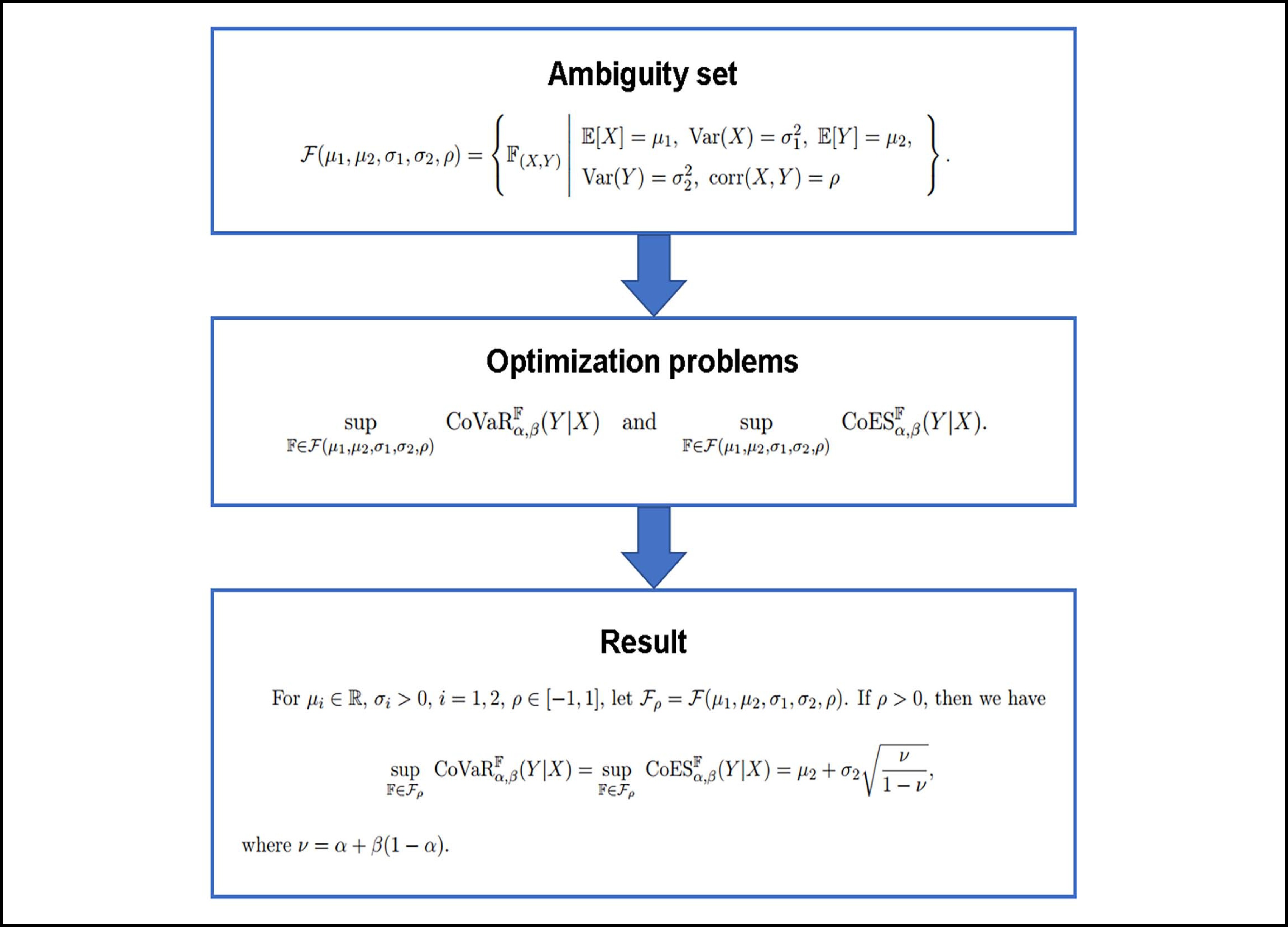
    We construct the above new ambiguity set, then propose the optimization problem of CoVaRand CoES based on this ambiguity set, and give the theoretical results.

    Public Summary

    • The relationship between CoVaR, CoES and dependence structure are investigated.
    • In case where the first two marginal moments are known, the closed-form solution and the valueof the worst-case CoVaR and CoES are derived.
    • The worst-case CoVaR and CoES under mean and covariance information are investigated.
    • FullText(HTML)
    References (18)
    Related Articles
    Supplements (1)
    Track Citations

Catalog

    Get Citation
    {{if article.pdfAccess}}
    {{if article.articleBusiness.pdfLink && article.articleBusiness.pdfLink != ''}} {{else}} {{/if}}PDF
    {{/if}}
    XML

    Figure  1.   CoVaR and CoES with bivariate Gaussian distributions.

    Figure  2.   CoVaR and CoES with bivariate t-distributions.

    Figure  3.   CoVaR and CoES with Pareto marginal and different copulas.

    [1]
    Basel Committee on Banking Supervision. Minimum capital requirements for market risk. Basel, Switzerland: Bank for International Settlements, 2019.
    [2]
    Adrian T, Brunnermeier M K. CoVaR. American Economic Review, 2016, 106 (7): 1705–1741. DOI: 10.1257/aer.20120555
    [3]
    Brownlees C, Engle R F. SRISK: A conditional capital shortfall measure of systemic risk. The Review of Financial Studies, 2017, 30 (1): 48–79. DOI: 10.1093/rfs/hhw060
    [4]
    Acharya V V, Pedersen L H, Philippon T, et al. Measuring systemic risk. The Review of Financial Studies, 2017, 30 (1): 2–47. DOI: 10.1093/rfs/hhw088
    [5]
    Girardi G, Ergün A T. Systemic risk measurement: Multivariate GARCH estimation of CoVaR. Journal of Banking & Finance, 2013, 37 (8): 3169–3180. DOI: 10.1016/j.jbankfin.2013.02.027
    [6]
    Huang W Q, Uryasev S. The CoCVaR approach: Systemic risk contribution measurement. Journal of Risk, 2018, 20 (4): 75–93. DOI: 10.21314/JOR.2018.383
    [7]
    López-Espinosa G, Moreno A, Rubia A, et al. Short-term wholesale funding and systemic risk: A global CoVaR approach. Journal of Banking & Finance, 2012, 36 (12): 3150–3162. DOI: 10.1016/j.jbankfin.2012.04.020
    [8]
    Karimalis E N, Nomikos N K. Measuring systemic risk in the European banking sector: A Copula CoVaR approach. The European Journal of Finance, 2018, 24 (11): 944–975. DOI: 10.1080/1351847X.2017.1366350
    [9]
    Popescu I. Robust mean-covariance solutions for stochastic optimization. Operations Research, 2007, 51 (1): 98–112. DOI: 10.1287/opre.1060.0353
    [10]
    Bertsimas D, Doan X V, Natarajan K, et al. Models for minimax stochastic linear optimization problems with risk aversion. Mathematics of Operations Research, 2010, 35 (3): 580–602. DOI: 10.1287/moor.1100.0445
    [11]
    Delage E, Ye Y. Distributionally robust optimization under moment uncertainty with application to data-driven problems. Operations Research, 2010, 58 (3): 595–612. DOI: 10.1287/opre.1090.0741
    [12]
    Natarajan K, Sim M, Uichanco J. Tractable robust expected utility and risk models for portfolio optimization. Mathematical Finance, 2010, 20 (4): 695–731. DOI: 10.1111/j.1467-9965.2010.00417.x
    [13]
    Wiesemann W, Kuhn D, Sim M. Distributionally robust convex optimization. Operations Research, 2014, 62 (6): 1358–1376. DOI: 10.1287/opre.2014.1314
    [14]
    Chen L, He S, Zhang S. Tight bounds for some risk measures, with applications to robust portfolio selection. Operations Research, 2011, 59 (4): 847–865. DOI: 10.1287/opre.1110.0950
    [15]
    Nelsen R B. An Introduction to Copulas. 2nd edition. New York: Springer, 2006.
    [16]
    Mainik G, Schaanning E. On dependence consistency of CoVaR and some other systemic risk measures. Statistics & Risk Modeling, 2014, 31 (1): 49–77. DOI: 10.1515/strm-2013-1164
    [17]
    Ghaoui L E, Oks M, Oustry F. Worst-case value-at-risk and robust portfolio optimization: A conic programming approach. Operations Research, 2003, 51 (4): 543–556. DOI: 10.1287/opre.51.4.543.16101
    [18]
    Bernard C, Pesenti S M, Vanduffel S. Robust distortion risk measures. https://ssrn.com/abstract=3677078 .

    Related Articles

    [1]Wenjun Yan, Jiaqi Zhang, Jianing Xu, Haonan He, Shanyong Wang. The optimization and selection of venture capital incentive policies under uncertain condition[J]. JUSTC, 2022, 52(8): 8-1-8-11. DOI: 10.52396/JUSTC-2022-0011
    [2]WEI Wenzhe, ZHOU Lei, WEI Jiuchang. Influence of firm crisis self-disclosure on stock reaction: Empirical research based on 220 safety accident announcements of Chinese A-share listed firms[J]. JUSTC, 2020, 50(8): 1035. DOI: 10.3969/j.issn.0253-2778.2020.08.001
    [3]KONG Haimei, LI Gen, SUN Liang. The uncertainty of projection of precipitation change in the middle and lower reaches of the Yangtze River under the RCP8.5 scenario[J]. JUSTC, 2020, 50(7): 1003. DOI: 10.3969/j.issn.0253-2778.2020.07.018
    [4]FEI Weiyin, YANG Shanshan, LIANG Yong. Continuous-time contracting problems with one-sided limited commitment under Knightian uncertainty[J]. JUSTC, 2020, 50(2): 146-155. DOI: 10.3969/j.issn.0253-2778.2020.02.010
    [5]ZHONG Bin, WANG Xinghu, SHENG Jie. Distributed adaptive control of nonlinear vehicular platoons[J]. JUSTC, 2019, 49(7): 588-594. DOI: 10.3969/j.issn.0253-2778.2019.07.009
    [6]CHEN Can, LIU Jie. Sellers optimal ordering quantity and pricing when considering consumer valuation bias[J]. JUSTC, 2015, 45(8): 692-701. DOI: 10.3969/j.issn.0253-2778.2015.08.011
    [7]JANG Shun, PAN Feng. Non-fragile stabilization for networked control systems with input delay and randomly occurring gain uncertainties[J]. JUSTC, 2015, 45(7): 614-622.
    [8]YU Minxiu, FEI Weiyin, LYU Huiying. Optimization of dynamic portfolio under model uncertainty[J]. JUSTC, 2014, 44(3): 194-202. DOI: 10.3969/j.issn.0253-2778.2014.03.005
    [9]SHI Xueqin, FEI Weiyin, HU Huimin, BAO Pinjuan. On optimal investment strategy of pension funds with a minimum guarantee under Knightian uncertainty[J]. JUSTC, 2014, 44(3): 188-193. DOI: 10.3969/j.issn.0253-2778.2014.03.004
    [10]HU Xinhan, YE Wuyi, MIAO Baiqi. Relationship between credit risk and market value of listed companies based on copula method[J]. JUSTC, 2013, 43(5): 410-419. DOI: 10.3969/j.issn.0253-2778.2013.05.009
    TrendMD
    2022 No. 5 Volume 52 Issue 5 PP. 4
    Cover

    Article Metrics

    Article views (907) PDF downloads (3111)

    Copyright © Editorial Office of JUSTC, All Rights Reserved. 皖ICP备05002528号

    Supported by: Beijing Renhe Information Technology Co., Ltd.  Baidu Analytics

    /

    DownLoad:  Full-Size Img  PowerPoint
    Return
    Return